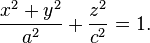Spheroid

From Wikipedia, the free encyclopedia


oblate spheroid	prolate spheroid

A spheroid, or ellipsoid of revolution is a quadric surface obtained by rotating an ellipse about one of its principal axes; in other words, an ellipsoid with two equal semi-diameters.

If the ellipse is rotated about its major axis, the result is a prolate (elongated) spheroid, like an American football or rugby ball. If the ellipse is rotated about its minor axis, the result is an oblate (flattened) spheroid, like a lentil. If the generating ellipse is a circle, the result is a sphere.

Because of the combined effects of gravitation and rotation, the Earth's shape is roughly that of a sphere slightly flattened in the direction of its axis. For that reason, in cartography the Earth is often approximated by an oblate spheroid instead of a sphere. The current World Geodetic System model uses a spheroid whose radius is 6,378.137 km at the equator and 6,356.752 km at the poles.

The word spheroid originally meant an approximately spherical body and that is how it is used in some older papers on geodesy. In order to avoid confusion, spheroid should be defined as an ellipsoid of revolution, if that is the intended meaning.

Equation

The assignment of semi-axes on a spheroid. Ii is oblate if c<a and prolate if c>a.

The equation of a tri-axial ellipsoid centred at the origin with semi-axes a,b, c aligned along the coordinate axes is

${\frac {x^{2}}{a^{2}}}+{\frac {y^{2}}{b^{2}}}+{\frac {z^{2}}{c^{2}}}=1$

The equation of a spheroid with Oz as the symmetry axis is given by setting a=b:

${\frac {x^{2}+y^{2}}{a^{2}}}+{\frac {z^{2}}{c^{2}}}=1.$

The semi-axis a is the equatorial radius of the spheroid, and c is the distance from centre to pole along the symmetry axis. There are two possible cases:

c < a : oblate spheroid
c > a : prolate spheroid

The case of a=c reduces to a sphere.

Surface area

An oblate spheroid with c < a has surface area

$S_{{{\rm {oblate}}}}=2\pi a^{2}\left(1+{\frac {1-e^{2}}{e}}\tanh ^{{-1}}e\right)\quad {\mbox{where}}\quad e^{2}=1-{\frac {c^{2}}{a^{2}}}.$

The oblate spheroid is generated by rotation about the Oz axis of an ellipse with semi-major axis a and semi-minor axis c, therefore e may be identified as the eccentricity. (See ellipse). A derivation of this result may be found at.^[1]

A prolate spheroid with c > a has surface area

$S_{{{\rm {prolate}}}}=2\pi a^{2}\left(1+{\frac {c}{ae}}\sin ^{{-1}}e\right)\qquad {\mbox{where}}\qquad e^{2}=1-{\frac {a^{2}}{c^{2}}}.$

The prolate spheroid is generated by rotation about the Oz axis of an ellipse with semi-major axis c and semi-minor axis a, therefore e may again be identified as the eccentricity. (See ellipse). A derivation of this result may be found at.^[2]

These formulas are identical in the sense that the formula for $S_{{{\rm {oblate}}}}$ can be used to calculate the surface area of a prolate spheroid and vice versa. However, e then becomes imaginary and can no longer directly be identified with the eccentricity. Both of these results may be cast into many other forms using standard mathematical identities and relations between parameters of the ellipse.

Volume

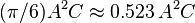The volume of a spheroid (of any kind) is $(4\pi /3)a^{2}c\approx 4.19\,a^{2}c$ . If A=2a is the equatorial diameter, and C=2c is the polar diameter, the volume is $(\pi /6)A^{2}C\approx 0.523\,A^{2}C$ .

Curvature

If a spheroid is parameterized as

${\vec \sigma }(\beta ,\lambda )=(a\cos \beta \cos \lambda ,a\cos \beta \sin \lambda ,c\sin \beta );\,\!$

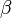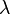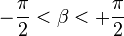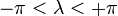where $\beta \,\!$ is the reduced or parametric latitude, $\lambda \,\!$ is the longitude, and $-{\frac {\pi }{2}}<\beta <+{\frac {\pi }{2}}\,\!$ and $-\pi <\lambda <+\pi \,\!$ , then its Gaussian curvature is

$K(\beta ,\lambda )={c^{2} \over (a^{2}+(c^{2}-a^{2})\cos ^{2}\beta )^{2}};\,\!$

and its mean curvature is

$H(\beta ,\lambda )={c(2a^{2}+(c^{2}-a^{2})\cos ^{2}\beta ) \over 2a(a^{2}+(c^{2}-a^{2})\cos ^{2}\beta )^{{3/2}}}.\,\!$

Both of these curvatures are always positive, so that every point on a spheroid is elliptic.

References

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.