Spherical cap

In geometry, a spherical cap or spherical dome is a portion of a sphere cut off by a plane. If the plane passes through the center of the sphere, so that the height of the cap is equal to the radius of the sphere, the spherical cap is called a hemisphere.
Volume and surface area
If the radius of the base of the cap is  , and the height of the cap is
, and the height of the cap is  , then the volume of the spherical cap is
, then the volume of the spherical cap is
and the curved surface area of the spherical cap is
The relationship between  and
and  is irrelevant as long as
is irrelevant as long as 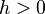 and
and 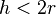 . The blue section of the illustration is also a spherical cap.
. The blue section of the illustration is also a spherical cap.
The parameters  ,
,  and
and  are not independent:
are not independent:
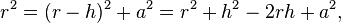
 .
.
Substituting this into the area formula gives:
Note also that in the upper hemisphere of the diagram,  , and in the lower hemisphere
, and in the lower hemisphere 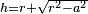 ; hence in either hemisphere
; hence in either hemisphere  and so an alternative expression for the volume is
and so an alternative expression for the volume is
 .
.
Application
The volume of all points which are in at least one of two intersecting spheres of radii r1 and r2 is [1]
 ,
,
where
is the total of the two isolated spheres, and
the sum of the two spherical caps of the intersection. If d <r1+r2 is the distance between the two sphere centers, elimination of the variables h1 and h2 leads to[2] [3]
Generalizations
Sections of other solids
The spheroidal dome is obtained by sectioning off a portion of a spheroid so that the resulting dome is circularly symmetric (having an axis of rotation), and likewise the ellipsoidal dome is derived from the ellipsoid.
Hyperspherical cap
Generally, the  -dimensional volume of a hyperspherical cap of height
-dimensional volume of a hyperspherical cap of height  and radius
and radius  in
in  -dimensional Euclidean space is given by [4]
-dimensional Euclidean space is given by [4]
where  (the gamma function) is given by
(the gamma function) is given by  .
.
The formula for  can be expressed in terms of the volume of the unit n-ball
can be expressed in terms of the volume of the unit n-ball ![C_{{n}}={\scriptstyle \pi ^{{n/2}}/\Gamma [1+{\frac {n}{2}}]}](/2014-wikipedia_en_all_02_2014/I/media/9/1/5/f/915fd60d8b00a6880264bbba9f90d9f3.png) and the hypergeometric function
and the hypergeometric function  or the regularized incomplete beta function
or the regularized incomplete beta function  as
as
![V=C_{{n}}\,r^{{n}}\left({\frac {1}{2}}\,-\,{\frac {r-h}{r}}\,{\frac {\Gamma [1+{\frac {n}{2}}]}{{\sqrt {\pi }}\,\Gamma [{\frac {n+1}{2}}]}}{\,\,}_{{2}}F_{{1}}\left({\tfrac {1}{2}},{\tfrac {1-n}{2}};{\tfrac {3}{2}};\left({\tfrac {r-h}{r}}\right)^{{2}}\right)\right)={\frac {1}{2}}C_{{n}}\,r^{n}I_{{(2rh-h^{2})/r^{2}}}\left({\frac {n+1}{2}},{\frac {1}{2}}\right)](/2014-wikipedia_en_all_02_2014/I/media/f/8/2/a/f82a83925d3303e7a29479f2f45801f5.png) ,
,
and the area formula  can be expressed in terms of the area of the unit n-ball
can be expressed in terms of the area of the unit n-ball ![A_{{n}}={\scriptstyle 2\pi ^{{n/2}}/\Gamma [{\frac {n}{2}}]}](/2014-wikipedia_en_all_02_2014/I/media/b/f/d/c/bfdcc6765cbc5944514eb03a3f32f3ed.png) as
as
 ,
,
where 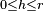 .
.
See also
- Circular segment — the analogous 2D object.
- Dome (mathematics)
- Solid angle — contains formula for n-sphere caps
- Spherical segment
- Spherical sector
- Spherical wedge
References
- ↑ Connolly, Michael L. (1985). "Computation of molecular volume". J. Am. Chem. Soc: 1118–1124. doi:10.1021/ja00291a006.
- ↑ Pavani, R.; Ranghino, G. (1982). "A method to compute the volume of a molecule". Comput. Chem. doi:10.1016/0097-8485(82)80006-5.
- ↑ Bondi, A. (1964). "van der Waals volumes and radii". J. Phys. Chem. (68): 441–451. doi:10.1021/j100785a001.
- ↑ Li, S. (2011). "Concise Formulas for the Area and Volume of a Hyperspherical Cap". Asian J. Math. Stat. 4 (1): 66–70. doi:10.3923/ajms.2011.66.70
- Richmond, Timothy J. (1984). "Solvent accessible surface area and excluded volume in proteins: Analytical equation for overlapping spheres and implications for the hydrophobic effect". J. Molec. Biol. 178 (1): 63–89. doi:10.1016/0022-2836(84)90231-6.
- Lustig, Rolf (1986). "Geometry of four hard fused spheres in an arbitrary spatial configuration". Mol. Phys. 59 (2): 195–207. Bibcode:1986MolPh..59..195L. doi:10.1080/00268978600102011.
- Gibson, K. D.; Scheraga, Harold A. (1987). "Volume of the intersection of three spheres of unequal size: a simplified formula". J. Phys. Chem. 91 (15): 4121–4122.
- Gibson, K. D.; Scheraga, Harold A. (1987). "Exact calculation of the volume and surface area of fused hard-sphere molecules with unequal atomic radii". Mol. Phys. 62 (5): 1247–1265. Bibcode:1987MolPh..62.1247G. doi:10.1080/00268978700102951.
- Petitjean, Michel (1994). "On the analytical calculation of van der Waals surfaces and volumes: some numerical aspects". Int. J. Quant. Chem. 15 (5): 507–523.
- Grant, J. A.; Pickup, B. T. (1995). "A Gaussian description of molecular shape". J. Phys. Chem. 99 (11): 3503–3510. doi:10.1021/j100011a016.
- Busa, Jan; Dzurina, Jozef; Hayryan, Edik; Hayryan, Shura (2005). "ARVO: A fortran package for computing the solvent accessible surface area and the excluded volume of overlapping spheres via analytic equations". Comp. Phys. Commun. 165: 59–96. Bibcode:2005CoPhC.165...59B. doi:10.1016/j.cpc.2004.08.002.
- Li, S. (2011). "Concise Formulas for the Area and Volume of a Hyperspherical Cap". Asian J. Math. Stat. 4 (1): 66–70. doi:10.3923/ajms.2011.66.70..
External links
| Wikimedia Commons has media related to Spherical caps. |
- Weisstein, Eric W., "Spherical cap", MathWorld., derivation and some additional formulas
- Online calculator for spherical cap volume and area
- Summary of spherical formulas

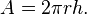


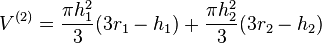
![V^{{(2)}}={\frac {\pi }{12d}}(r_{1}+r_{2}-d)^{2}[d^{2}+2d(r_{1}+r_{2})-3(r_{1}-r_{2})^{2}].](/2014-wikipedia_en_all_02_2014/I/media/8/4/7/2/8472d0e17d7346b4b30b86b1605479b8.png)
