Solow–Swan model
The Solow–Swan model, also known as exogenous growth model, is an economic model of long-run economic growth set within the framework of neoclassical economics. It attempts to explain long-run economic growth by looking at productivity, capital accumulation, population growth, and technological progress. At its core is the neoclassical aggregate production function of Cobb–Douglas type, which enables the model “to make contact with microeconomics”.[1]:26 The model was developed independently by Robert Solow and Trevor Swan in 1956,[2][3] and superseded the post-Keynesian Harrod–Domar model. Due to its simplicity, Solow–Swan proved to be a valid starting point for various extensions. For instance, in 1965, David Cass and Tjalling Koopmans integrated Frank Ramsey's analysis of consumer optimization, thereby endogenizing the savings rate—see the Ramsey–Cass–Koopmans model.
Background
The neo-classical model was an extension to the 1946 Harrod–Domar model that included a new term: productivity growth. Important contributions to the model came from the work done by Solow and by Swan in 1956, who independently developed relatively simple growth models.[2][3] Solow's model fitted available data on US economic growth with some success.[4] In 1987 Solow was awarded the Nobel Prize in Economics for his work. Solow was the first economist to develop a growth model which distinguished between vintages of capital.[5] In Solow's model, new capital is more valuable than old (vintage) capital because—since capital is produced based on known technology, and technology improves with time—new capital will be more productive than old capital.[5] Both Paul Romer and Robert Lucas, Jr. subsequently developed alternatives to Solow's neo-classical growth model.[5] Today, economists use Solow's sources-of-growth accounting to estimate the separate effects on economic growth of technological change, capital, and labor.[5]
Extension to the Harrod–Domar model
Solow extended the Harrod–Domar model by:
- Adding labor as a factor of production;
- And capital-labor ratios are not fixed as they are in the Harrod–Domar model. These refinements allow increasing capital intensity to be distinguished from technological progress.
Short-run implications
- Policy measures like tax cuts or investment subsidies can affect the steady state level of output but not the long-run national curve.
- Growth is affected only in the short-run as the economy converges to the new steady state output level.
- The rate of growth as the economy converges to the steady state is determined by the rate of capital accumulation.
- Capital accumulation is in turn determined by the savings rate (the proportion of output used to create more capital rather than being consumed) and the rate of capital depreciation.
Long-run implications
In neoclassical growth models, the long-run rate of growth is exogenously determined – in other words, it is determined outside of the model. A common prediction of these models is that an economy will always converge towards a steady state rate of growth, which depends only on the rate of technological progress and the rate of labor force growth.
A country with a higher saving rate will experience faster growth, e.g. Singapore had a 40% saving rate in the period 1960 to 1996 and annual GDP growth of 5-6%, compared with Kenya in the same time period which had a 15% saving rate and annual GDP growth of just 1%. This relationship was anticipated in the earlier models, and is retained in the Solow model; however, in the very long-run, capital accumulation appears to be less significant than technological innovation in the Solow model.
Assumptions
The key assumption of the neoclassical growth model is that capital is subject to diminishing returns in a closed economy.
→Given a fixed stock of labor, the impact on output of the last unit of capital accumulated will always be less than the one before.
→Assuming for simplicity no technological progress or labor force growth, diminishing returns implies that at some point the amount of new capital produced is only just enough to make up for the amount of existing capital lost due to depreciation. At this point, because of the assumptions of no technological progress or labor force growth, the economy ceases to grow.
→Assuming non-zero rates of labor growth complicates matters somewhat, but the basic logic still applies – in the short-run the rate of growth slows as diminishing returns take effect and the economy converges to a constant "steady-state" rate of growth (that is, no economic growth per-capita).
→Including non-zero technological progress is very similar to the assumption of non-zero workforce growth, in terms of "effective labor": a new steady state is reached with constant output per worker-hour required for a unit of output. However, in this case, per-capita output is growing at the rate of technological progress in the "steady-state" (that is, the rate of productivity growth).
Variations in the effects of productivity
Within the Solow growth model, the Solow residual or total factor productivity is an often used measure of technological progress. The model can be reformulated in slightly different ways using different productivity assumptions, or different measurement metrics:
- Average Labor Productivity (ALP) is economic output per labor hour.
- Multifactor productivity (MFP) is output divided by a weighted average of capital and labor inputs. The weights used are usually based on the aggregate input shares either factor earns. This ratio is often quoted as: 33% return to capital and 66% return to labor (in Western nations), but Robert J. Gordon says the weight to labor is more commonly assumed to be 75%.
In a growing economy, capital is accumulated faster than people are born, so the denominator in the growth function under the MFP calculation is growing faster than in the ALP calculation. Hence, MFP growth is almost always lower than ALP growth. (Therefore, measuring in ALP terms increases the apparent capital deepening effect.) MFP is measured by the "Solow residual", not ALP.
Solow–Swan model
The textbook Solow–Swan model is set in continuous-time world with no government or international trade. A single good (output) is produced—using two essential factors of production, labour ( ) and capital (
) and capital ( )—with an aggregate production function that satisfies the Inada conditions, which implies it must be asymptotically Cobb–Douglas.[6]
)—with an aggregate production function that satisfies the Inada conditions, which implies it must be asymptotically Cobb–Douglas.[6]
where 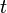 denotes time,
denotes time,  is production elasticity as well as capital to income ratio, and
is production elasticity as well as capital to income ratio, and  represents the total production.
represents the total production.  refers to labour-augmenting technology or “knowledge”, thus
refers to labour-augmenting technology or “knowledge”, thus 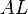 represents effective labour. All factors of production are fully employed, and initial values
represents effective labour. All factors of production are fully employed, and initial values 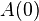 ,
, 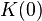 , and
, and 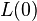 are given. The number of workers, i.e. labour, as well as the level of technology exogenously grow in time at rates
are given. The number of workers, i.e. labour, as well as the level of technology exogenously grow in time at rates  and
and 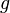 , respectively:
, respectively:
The number of effective units of labour, 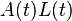 , therefore grows at rate
, therefore grows at rate 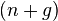 . Meanwhile, the stock of capital depreciates over time at a constant rate
. Meanwhile, the stock of capital depreciates over time at a constant rate  . However, only a fraction of the output (
. However, only a fraction of the output (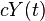 with
with  ) is consumed, leaving a saved share of
) is consumed, leaving a saved share of  for investment:
for investment:
where  is the constant savings rate, and
is the constant savings rate, and 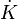 is shorthand for
is shorthand for 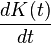 , the derivative with respect to time.
, the derivative with respect to time.
Since the production function 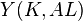 has constant returns to scale, it can be written in terms per effective unit of labour:[note 1]
has constant returns to scale, it can be written in terms per effective unit of labour:[note 1]
The main interest of the model is the dynamics of capital intensity  , the capital stock per unit of effective labour. Its behaviour over time is given by the key equation of the Solow–Swan model:[note 2]
, the capital stock per unit of effective labour. Its behaviour over time is given by the key equation of the Solow–Swan model:[note 2]
The first term, 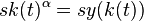 , is the actual investment per unit of effective labour: the fraction
, is the actual investment per unit of effective labour: the fraction  of the output per unit of effective labour
of the output per unit of effective labour  that is saved and invested. The second term,
that is saved and invested. The second term, 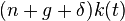 , is the “break-even investment”: the amount of investment that must be invested to prevent
, is the “break-even investment”: the amount of investment that must be invested to prevent  from falling.[7]:16 The equation implies that
from falling.[7]:16 The equation implies that  converges to a steady-state value of
converges to a steady-state value of  , defined by
, defined by 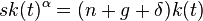 , at which there is neither increase nor decrease of capital intensity:
, at which there is neither increase nor decrease of capital intensity:
at which the stock of capital  and effective labour
and effective labour 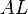 are growing at rate
are growing at rate 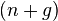 . By assumption of constant returns, output
. By assumption of constant returns, output  is also growing at that rate. In essence, the Solow–Swan model predicts that an economy will converge to a balanced-growth equilibrium, regardless of its starting point. In this situation, the growth of output per worker is determined solely by the rate of technological progress.[7]:18
is also growing at that rate. In essence, the Solow–Swan model predicts that an economy will converge to a balanced-growth equilibrium, regardless of its starting point. In this situation, the growth of output per worker is determined solely by the rate of technological progress.[7]:18
Mankiw–Romer–Weil model
After empirically testing the Solow–Swan model, N. Gregory Mankiw, David Romer, and David Weil suggested a human capital augmented version of the model that is more consistent with actual data.[8] Similar to the textbook Solow–Swan model, the production function is of Cobb–Douglas type:
 ,
,
where 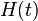 is the stock of human capital, which depreciates at the same rate
is the stock of human capital, which depreciates at the same rate  as physical capital. For simplicity, they assume the same function of accumulation for both types of capital. Like in Solow–Swan, a fraction of the outcome,
as physical capital. For simplicity, they assume the same function of accumulation for both types of capital. Like in Solow–Swan, a fraction of the outcome, 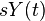 , is saved each period, but in this case split up and invested partly in physical and partly in human capital, such that
, is saved each period, but in this case split up and invested partly in physical and partly in human capital, such that  . Therefore, there are two fundamental dynamic equations in this model:
. Therefore, there are two fundamental dynamic equations in this model:
The balanced (or steady-state) equilibrium growth path is determined by  , which means
, which means  and
and  . Solving for the optimal level of
. Solving for the optimal level of  and
and  yields:
yields:
Both values define optimum per capita growth,  .
.
Empirical evidence
A key prediction of neoclassical growth models is that the income levels of poor countries will tend to catch up with or converge towards the income levels of rich countries as long as they have similar characteristics – for instance saving rates. Since the 1950s, the opposite empirical result has been observed on average. If the average growth rate of countries since, say, 1960 is plotted against initial GDP per capita (i.e. GDP per capita in 1960), one observes a positive relationship. In other words, the developed world appears to have grown at a faster rate than the developing world, the opposite of what is expected according to a prediction of convergence. However, a few formerly poor countries, notably Japan, do appear to have converged with rich countries, and in the case of Japan actually exceeded other countries' productivity, some theorize that this is what has caused Japan's poor growth recently – convergent growth rates are still expected, even after convergence has occurred; leading to over-optimistic investment, and actual recession.
The evidence is stronger for convergence within countries. For instance the per-capita income levels of the southern states of the United States have tended to converge to the levels in the Northern states. These observations have led to the adoption of the conditional convergence concept. Whether convergence occurs or not depends on the characteristics of the country or region in question, such as:
- Institutional arrangements
- Free markets internally, and trade policy with other countries.
- Education policy
Evidence for conditional convergence comes from multivariate, cross-country regressions.
If productivity were associated with high technology then the introduction of information technology should have led to a noticeable productivity acceleration over the past twenty years; but it has not: see: Solow computer paradox.
Econometric analysis on Singapore and the other "East Asian Tigers" has produced the surprising result that although output per worker has been rising, almost none of their rapid growth had been due to rising per-capita productivity (they have a low "Solow residual").
See also
- Economic growth
- Endogenous growth theory
- Golden rule savings rate
Notes
References
- ↑ Acemoglu, Daron (2009). "The Solow Growth Model". Introduction to Modern Economic Growth. Princeton: Princeton University Press. pp. 26–76. ISBN 978-0-691-13292-1.
- ↑ 2.0 2.1 Solow, Robert M. (1956). "A Contribution to the Theory of Economic Growth". Quarterly Journal of Economics 70 (1): 65–94. doi:10.2307/1884513.
- ↑ 3.0 3.1 Swan, Trevor W. (1956). "Economic Growth and Capital Accumulation". Economic Record 32 (2): 334–361. doi:10.1111/j.1475-4932.
- ↑ Solow, Robert M. (1957). "Technical Change and the Aggregate Production Function". Review of Economics and Statistics 39 (3): 312–320. doi:10.2307/1926047.
- ↑ 5.0 5.1 5.2 5.3 Haines, Joel D. (January 1, 2006) Competitiveness Review A framework for managing the sophistication of the components of technology for global competition. Volume 16; Issue 2; Page 106.
- ↑ Barelli, Paulo; Pessôa, Samuel de Abreu (2003). "Inada conditions imply that production function must be asymptotically Cobb–Douglas". Economics Letters 81 (3): 361–363. doi:10.1016/S0165-1765(03)00218-0.
- ↑ 7.0 7.1 Romer, David (2011). "The Solow Growth Model". Advanced Macroeconomics (Fourth ed.). New York: McGraw-Hill. pp. 6–48. ISBN 978-0-07-351137-5.
- ↑ Mankiw, N. Gregory; Romer, David; Weil, David N. (May 1992). "A Contribution to the Empirics of Economic Growth". The Quarterly Journal of Economics 107 (2): 407–437. doi:10.2307/2118477. JSTOR 2118477.
Further reading
- Agénor, Pierre-Richard (2004). "Growth and Technological Progress: The Solow–Swan Model". The Economics of Adjustment and Growth (Second ed.). Cambridge: Harvard University Press. pp. 439–462. ISBN 0-674-01578-9.
- Barro, Robert J. (2008). "Working with the Solow Growth Model". Macroeconomics: A Modern Approach (Internat. student ed.). Mason, OH: Thomson South-Western. pp. 68–94. ISBN 978-0-324-54567-8.
- Barro, Robert J.; Sala-i-Martin, Xavier (2004). "Growth Models with Exogenous Saving Rates". Economic Growth (Second ed.). New York: McGraw-Hill. pp. 23–84. ISBN 0-262-02553-1.
- Farmer, Roger E. A. (1999). "Neoclassical Growth Theory". Macroeconomics (Second ed.). Cincinnati: South-Western. pp. 333–355. ISBN 0-324-12058-3.
External links
- Solow Model Videos - 20+ videos walking through derivation of the Solow Growth Model's Conclusions
- Java applet where you can experiment with parameters and learn about Solow model
- Solow Growth Model by Fiona Maclachlan, The Wolfram Demonstrations Project.
- A step-by-step explanation of how to understand the Solow Model
- Professor José-Víctor Ríos-Rull's course at University of Minnesota
- Professor Alex Tabarrok's Solow Growth Model lecture at MRUniversity
| |||||||||||||||||||||||


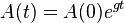

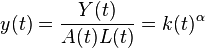


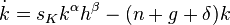

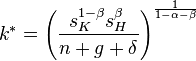


![{\dot {k}}(t)={\frac {{\dot {K}}(t)}{A(t)L(t)}}-{\frac {K(t)}{[A(t)L(t)]^{2}}}[A(t){\dot {L}}(t)+L(t){\dot {A}}(t)]={\frac {{\dot {K}}(t)}{A(t)L(t)}}-{\frac {K(t)}{A(t)L(t)}}{\frac {{\dot {L}}(t)}{L(t)}}-{\frac {K(t)}{A(t)L(t)}}{\frac {{\dot {A}}(t)}{A(t)}}](/2014-wikipedia_en_all_02_2014/I/media/e/6/a/0/e6a0fcb5645c4699a96ddfcc8682764c.png) . Since
. Since 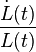 ,
, 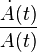 are
are 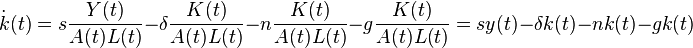 . As mentioned above,
. As mentioned above, 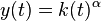 .
.