Singapore math
Singapore math (or Singapore maths in British English[1]) is a teaching method based on the national math curriculum used for kindergarten through sixth grade in Singapore.[2][3] It involves teaching students to learn and master fewer mathematical concepts at greater detail as well as having them learn these concepts using a three-step learning process.[2][3] The three steps are concrete, pictorial, and abstract. In the concrete step, students engage in hands-on learning experiences using concrete objects such as chips, dice, or paper clips.[4] This is followed by drawing pictorial representations of mathematical concepts. Students then solve mathematical problems in an abstract way by using numbers and symbols.[5]
The development of Singapore math began in the 1980s when the country's Ministry of Education developed its own mathematics textbooks that focused on problem solving and heuristic model drawing.[3][6] Outside Singapore, these textbooks were adopted by several schools in the the United States (U.S.) and in other countries such as Canada, Israel, and the United Kingdom.[1][7][8] Early adopters of these textbooks in the U.S. included parents interested in homeschooling as well as a limited number of schools.[3] These textbooks became more popular since the release of scores from the Trends in International Mathematics and Science Study (TIMSS), which showed Singapore at the top of the world three times in fourth and eighth grade mathematics.[9] U.S. editions of these textbooks have since been adopted by a large number of school districts as well as charter and private schools.[3]
History
Before the development of its own mathematics textbooks in the 1980s, Singapore imported its mathematics textbooks from other countries.[10] In 1981, the Curriculum Development Institute of Singapore (CDIS) (currently the Curriculum Planning and Development Division) began to develop its own mathematics textbooks and curriculum.[11] The CDIS developed and distributed a textbook series for elementary schools in Singapore called Primary Mathematics, which was first published in 1982 and subsequently revised in 1992 to emphasize problem solving.[11][12] In the late 1990s, the country's Ministry of Education opened the elementary school textbook market to private companies, and Marshall Cavendish, a local and private publisher of educational materials, began to publish and market the Primary Mathematics textbooks.[1][12][13]
Following Singapore’s curricular and instructional initiatives, dramatic improvements in math proficiency among Singaporean students on international assessments were observed.[1] TIMSS, an international assessment for math and science among fourth and eighth graders, ranked Singapore’s fourth and eighth grade students first in mathematics three times (1995, 1999, and 2003) among participating nations.[9][11] Likewise, the Organisation for Economic Co-operation and Development (OECD)'s Programme for International Student Assessment (PISA), a worldwide study of 15-year-old school students' scholastic performance in mathematics, science, and reading, has placed Singaporean students at second place, after Shanghai, China in 2009 and 2012.[14][15]
Since the TIMSS publication of Singapore's high ranking in mathematics, professional mathematicians in the U.S. took a closer look at Singapore mathematics textbooks such as Primary Mathematics.[9] In 2005, the American Institutes for Research (AIR) published a study, which concluded that U.S. schools could benefit from adopting these textbooks.[9] The textbooks were already distributed in the U.S. by Singapore Math, Inc., a private venture based in Oregon.[11] Early users of these textbooks in the U.S. included parents interested in homeschooling as well as a limited number of schools.[3] They became more popular since the release of the TIMSS scores showing Singapore's top ranking.[9] As of 2004, U.S. versions of Singapore mathematics textbooks were adopted in over 200 U.S. schools.[3][7] Schools and counties that had adopted these textbooks reported improvements in their students' performance.[7][9][13][16] Singapore math textbooks were also used in schools from other countries such as Canada, Israel, and the United Kingdom.[1][7][8]
Features
Covers fewer topics in greater depth
In contrast to a traditional U.S. math curriculum, Singapore math focuses students to learn fewer topics but at greater detail.[3] Each semester-level Singapore math textbook builds upon preceding levels of knowledge and skills, with students learning them to mastery before moving on to the next grade. This in turn prevents the need to reteach these skills to students at the next grade level.[2] By the end of sixth grade, Singapore math students would have mastered multiplication and division of fractions and are comfortable doing difficult multi-step word problems.[17]
In the U.S., it was found that Singapore math emphasizes the essential math skills recommended in the 2006 Focal Points publication by the National Council of Teachers of Mathematics (NCTM), the 2008 final report by the National Mathematics Advisory Panel, and the proposed Common Core State Standards, though it generally progresses to topics at an earlier grade level than indicated by those U.S. standards.[18][19]
Three-step learning process

Singapore math teaches students mathematical concepts in a three-step learning process: concrete, pictorial, and abstract.[3] This learning process was based on the work of an American Psychologist, Jerome Bruner. In the 1960s, Bruner found that people learn in three stages by first handling real objects before transitioning to pictures and then to symbols.[20] The Singapore government later adapted this approach to their math curriculum in the 1980s.
The first step of the three-step learning process is the concrete step, whereby students engage in hands-on learning experiences using concrete or real-world objects such as chips, dice, or paper clips.[4] Students would learn to count these objects (e.g., paper clips) by physically lining them up in a row. They would then learn basic arithmetic operations such as addition or subtraction by physically adding or removing the objects from each row.[20]
Students then transition to the second or pictorial step by drawing diagrams called "bar-models" to represent specific quantities of an object.[9][20] This involves drawing a rectangular bar to represent a specific quantity. For instance, one short bar would represent five paper clips whereas another bar that is twice as long would represent ten paperclips. By visualizing the difference between the two bars, students could learn to solve problems of addition by adding one bar to the other, which would, in this instance, produce an answer of fifteen paper clips. They can use this method to solve other mathematical problems involving subtraction, multiplication, and division.[9][17] As a tool, bar modeling is considered more efficient than the "guess-and-check" approach, whereby students try a combination of numbers until they found the right numbers that satisfy the conditions of a problem.[9]
Once students have learned to solve mathematical problems using bar modeling, they would transition to the third step by solving mathematical problems in an abstract way using numbers and symbols.
Bar modeling
Bar modeling is a pictorial method used to solve word problems in arithmetic.[17][21] These bar models can come in multiple forms such as a whole-part or a comparison model.
With the whole-part model, students would draw a rectangular bar to represent a "whole" larger quantity, which can be subdivided into two or more "parts." A student could be exposed to a word problem involving addition such as:
- If John has 70 apples and Jane has 30 apples, how many apples do they both have?
The solution to this problem could solved by drawing one bar would and dividing it into two parts, with the longer part as 70 and the shorter part as 30. By visualizing these two parts, students would simply solve the above word problem by adding both parts together to build a whole bar of 100. Conversely, a student could use whole-part model to solve a subtraction problem such as 100 - 70, by having the longer part be 70 and the whole bar be 100. They would then solve the problem by inferring the shorter part to be 30.
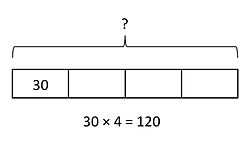
The whole-part model can also be used to solve problems involving multiplication or division.[22] A multiplication problem could be presented as follows:
- How much money would Jane have if she saved $30 each week for 4 weeks in a row?
The student could solve this multiplication problem by drawing one bar to represent the unknown answer, and subdivide that bar into four equal parts, with each part representing $30. Based on the drawn model, the student could then visualize this problem as providing a solution of $120.
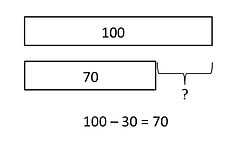
Unlike the whole-part model, a comparison model involves comparing two bars of unequal lengths.[17][21] It can be used to solve a subtraction problem such as the following:
- John needs to walk 100 miles to reach his home. So far, he has walked 70 miles. How many miles does he have left to walk home?
By using the comparison model, the student would draw one long bar to represent 100 and another shorter bar to represent 70. By comparing these two bars, students could then solve for the difference between the two numbers, which in this case is 30 miles. Like the whole-part model, the subtraction model can also be used to solve word problems involving addition, multiplication, and division.
See also
References
- ↑ 1.0 1.1 1.2 1.3 1.4 The Independent (July 2, 2009). "Box clever: Singapore's magic formula for maths success". The Independent.
- ↑ 2.0 2.1 2.2 Brown, Laura L. "What’s Singapore Math?". PBS. Retrieved September 19, 2013.
- ↑ 3.0 3.1 3.2 3.3 3.4 3.5 3.6 3.7 3.8 Hu, Winnie (September 30, 2010). "Making Math Lessons as Easy as 1, Pause, 2, Pause ...". The New York Times (New York, NY).
- ↑ 4.0 4.1 Knake, Lindsay (December 2011). "Saginaw Township elementary schools implement hands-on Singapore math program". MLive (Grand Rapids, MI).
- ↑ Jackson, Bill (October 10, 2012). "My view: America's students can benefit from Singapore math". CNN (Atlanta, GA).
- ↑ Wright, Gerard (Mat 12, 2008). "Mathematics Mighty Ducks". The Age (Australia).
- ↑ 7.0 7.1 7.2 7.3 Prystay, Cris (December 13, 2004). "As math skills slip, U.S. schools seek answers from Asia". The Wall Street Journal.
- ↑ 8.0 8.1 Wong, Khoon Yoong; Lee, Ngan Hoe (February 19, 2009). "Singapore education and mathematics curriculum". In Wong Koon Yoong; Lee Peng Yee; Berinderjeet Kaur; Foong Pui Yee; Ng Swee Fong. Mathematics Education: The Singapore Journey 2. Singapore: World Scientific Publishing. pp. 13–47. ISBN 9-812-83375-7.
- ↑ 9.0 9.1 9.2 9.3 9.4 9.5 9.6 9.7 9.8 Garelick, Barry (Fall 2006). "Miracle math: A successful program from Singapore tests the limits of school reform in the suburbs.". Educational Next 6.
- ↑ Lee, Peng Yee (September 12, 2008). "Sixty years of mathematics syllabi and textbooks in Singapore". In Usiskin, Zalman; Willmore, Edwin. Mathematics Curriculum in Pacific Rim Countries--China, Japan, Korea, and Singapore Proceedings of a Conference. Information Age Publishing. pp. 85–92. ISBN 1-593-11953-4.
- ↑ 11.0 11.1 11.2 11.3 Garelick, Barry (2006). "A tale of two countries and one school district.". Nonpartisan Education Review 6 (8).
- ↑ 12.0 12.1 Fang, Yanping; Lee, Christine Kim-Eng; Haron, Sharifah Thalha Bte Syed (February 19, 2009). "Lesson study in mathematics: Three cases in Singapore". In Wong Koon Yoong; Lee Peng Yee; Berinderjeet Kaur; Foong Pui Yee; Ng Swee Fong. Mathematics Education: The Singapore Journey 2. Singapore: World Scientific Publishing. pp. 104–129. ISBN 9-812-83375-7.
- ↑ 13.0 13.1 Landsberg, Mitchell (March 9, 2008). "In L.A., Singapore math has added value". Los Angeles Times (Los Angeles, CA).
- ↑ Dillon, sam (December 7, 2010). "Top test scores from shanghai stun educators". New York Times (New York, NY).
- ↑ The Economist (December 7, 2013). "Finn-ished". The Economist.
- ↑ Moroney, Kyle (December 2, 2013). "How Common Core standards are affecting elementary, middle school math classes". MLive.
- ↑ 17.0 17.1 17.2 17.3 Hoven, John; Garelick, Barry (November 2007). "Singapore Math: Using the bar model approach, Singapore textbooks enable students to solve difficult math problems--and learn how to think symbolically.". Educational Leadership 65: 38–21.
- ↑ National Mathematics Advisory Panel (March 2008). "Foundations for Success: The Final Report of the National Mathematics Advisory Panel". U.S. Department of Education. Retrieved December 13, 2013.
- ↑ Garland, Sarah (October 16, 2013). "How Does Common Core Compare?". Huffington Post.
- ↑ 20.0 20.1 20.2 BBC (December 2, 2013). "Can the Singapore method help your children learn maths?". BBC.
- ↑ 21.0 21.1 Frank Schaffer Publications. "Introduction to Singapore Math". 70 Must-Know Word Problems, Grade 7 (Singapore Math) (workbook ed.). Frank Schaffer Publications. pp. 3–8. ISBN 0-768-24016-6.
- ↑ Jackson, Bill. "Singapore math bar model strategy". The Daily Riff. Retrieved December 16, 2013.
External links
- BBC (December 2, 2013). "Can the Singapore method help your children learn maths?". BBC.
- Jackson, B. "Singapore Math Demystified!". The Daily Riff. Retrieved September 20, 2013.
| ||||||||