Serpentine curve
From Wikipedia, the free encyclopedia
A serpentine curve is a curve whose equation is of the form
 , where
, where 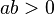 . Equivalently, it has a parametric representation
. Equivalently, it has a parametric representation 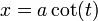 ,
,  , or functional representation
, or functional representation 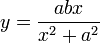 . Serpentine curves were studied by L'Hôpital and Huygens, and named and classified by Newton.
. Serpentine curves were studied by L'Hôpital and Huygens, and named and classified by Newton.
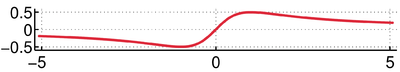
The serpentine curve for 

External links
This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.