Secondary polynomials

From Wikipedia, the free encyclopedia

In mathematics, the secondary polynomials 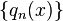$\{q_{n}(x)\}$ associated with a sequence $\{p_{n}(x)\}$ of polynomials orthogonal with respect to a density $\rho (x)$ are defined by

$q_{n}(x)=\int _{{\mathbb {R}}}\!{\frac {p_{n}(t)-p_{n}(x)}{t-x}}\rho (t)\,dt.$

To see that the functions 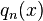$q_{n}(x)$ are indeed polynomials, consider the simple example of 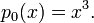$p_{0}(x)=x^{3}.$ Then,

${\begin{aligned}q_{0}(x)&{}=\int _{{\mathbb {R}}}\!{\frac {t^{3}-x^{3}}{t-x}}\rho (t)\,dt\\&{}=\int _{{\mathbb {R}}}\!{\frac {(t-x)(t^{2}+tx+x^{2})}{t-x}}\rho (t)\,dt\\&{}=\int _{{\mathbb {R}}}\!(t^{2}+tx+x^{2})\rho (t)\,dt\\&{}=\int _{{\mathbb {R}}}\!t^{2}\rho (t)\,dt+x\int _{{\mathbb {R}}}\!t\rho (t)\,dt+x^{2}\int _{{\mathbb {R}}}\!\rho (t)\,dt\end{aligned}}$

which is a polynomial $x$ provided that the three integrals in 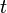$t$ (the moments of the density $\rho$ ) are convergent.

Secondary polynomials

See also