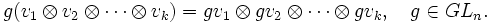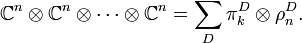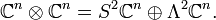Schur–Weyl duality

From Wikipedia, the free encyclopedia

Schur–Weyl duality is a mathematical theorem in representation theory that relates irreducible finite-dimensional representations of the general linear and symmetric groups. It is named after two pioneers of representation theory of Lie groups, Issai Schur, who discovered the phenomenon, and Hermann Weyl, who popularized it in his books on quantum mechanics and classical groups as a way of classifying representations of unitary and general linear groups.

Description

Schur–Weyl duality forms an archetypical situation in representation theory involving two kinds of symmetry that determine each other. Consider the tensor space

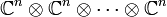${\mathbb {C}}^{n}\otimes {\mathbb {C}}^{n}\otimes \cdots \otimes {\mathbb {C}}^{n}$ with k factors.

The symmetric group S_k on k letters acts on this space (on the left) by permuting the factors,

$\sigma (v_{1}\otimes v_{2}\otimes \cdots \otimes v_{k})=v_{{\sigma ^{{-1}}(1)}}\otimes v_{{\sigma ^{{-1}}(2)}}\otimes \cdots \otimes v_{{\sigma ^{{-1}}(k)}}.$

The general linear group GL_n of invertible n×n matrices acts on it by the simultaneous matrix multiplication,

$g(v_{1}\otimes v_{2}\otimes \cdots \otimes v_{k})=gv_{1}\otimes gv_{2}\otimes \cdots \otimes gv_{k},\quad g\in GL_{n}.$

These two actions commute, and in its concrete form, the Schur–Weyl duality asserts that under the joint action of the groups S_k and GL_n, the tensor space decomposes into a direct sum of tensor products of irreducible modules for these two groups that determine each other,

${\mathbb {C}}^{n}\otimes {\mathbb {C}}^{n}\otimes \cdots \otimes {\mathbb {C}}^{n}=\sum _{D}\pi _{k}^{D}\otimes \rho _{n}^{D}.$

The summands are indexed by the Young diagrams D with k boxes and at most n rows, and representations 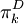$\pi _{k}^{D}$ of S_k with different D are mutually non-isomorphic, and the same is true for representations $\rho _{n}^{D}$ of GL_n.

The abstract form of the Schur–Weyl duality asserts that two algebras of operators on the tensor space generated by the actions of GL_n and S_k are the full mutual centralizers in the algebra of the endomorphisms 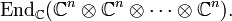${\mathrm {End}}_{{\mathbb {C}}}({\mathbb {C}}^{n}\otimes {\mathbb {C}}^{n}\otimes \cdots \otimes {\mathbb {C}}^{n}).$

Example

Suppose that k = 2 and n is greater than one. Then the Schur–Weyl duality is the statement that the space of two-tensors decomposes into symmetric and antisymmetric parts, each of which is an irreducible module for GL_n:

${\mathbb {C}}^{n}\otimes {\mathbb {C}}^{n}=S^{2}{\mathbb {C}}^{n}\oplus \Lambda ^{2}{\mathbb {C}}^{n}.$

The symmetric group S₂ consists of two elements and has two irreducible representations, the trivial representation and the sign representation. The trivial representation of S₂ gives rise to the symmetric tensors, which are invariant (i.e. do not change) under the permutation of the factors, and the sign representation corresponds to the skew-symmetric tensors, which flip the sign.

References

Roger Howe, Perspectives on invariant theory: Schur duality, multiplicity-free actions and beyond. The Schur lectures (1992) (Tel Aviv), 1–182, Israel Math. Conf. Proc., 8, Bar-Ilan Univ., Ramat Gan, 1995. MR 1321638
Issai Schur, Über eine Klasse von Matrizen, die sich einer gegebenen Matrix zuordnen lassen. Dissertation. Berlin. 76 S (1901) JMF 32.0165.04
Issai Schur, Über die rationalen Darstellungen der allgemeinen linearen Gruppe. Sitzungsberichte Akad. Berlin 1927, 58–75 (1927) JMF 53.0108.05
Hermann Weyl, The Classical Groups. Their Invariants and Representations. Princeton University Press, Princeton, N.J., 1939. xii+302 pp. MR 0000255

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.