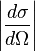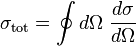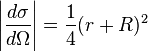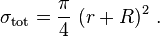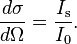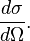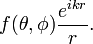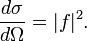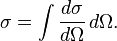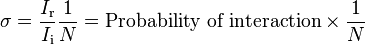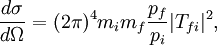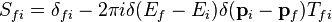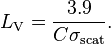Scattering cross-section

From Wikipedia, the free encyclopedia

The scattering cross-section is a hypothetical area which describes the likelihood of light or other radiation being scattered by a particle, the scattering center. It is a measure of the strength of the interaction between the scattered particle and one or several scattering centers.

Definition

In the simplest case, the differential scattering cross section refers to the way a single particle is scattered on a single scattering center:

The impact parameter is the perpendicular offset of the trajectory of the incoming particle. The differential of the cross section is the area element in the plane of the impact parameter, i.e. 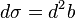$d\sigma =d^{2}b$ , where $b$ is the impact parameter. The differential cross section is the differential quotient of this area element by the solid angle element in the direction of the particle exit trajectory:

$\left|{\frac {d\sigma }{d\Omega }}\right|$

It describes the change in the impact parameter necessary to cause a given change in the exit trajectory direction. The definition is slightly counterintuitive in that the independent variable (in the denominator) describes the effect and the dependent variable (in the numerator) the initial condition. The differential cross section is always taken to be positive, even though in the most frequent case of limited-range repulsive interactions, larger impact parameters cause less deflection. In rotationally symmetric problems, the azimuthal angle $\varphi$ is not changed by the scattering process, and the differential cross section becomes

${\frac b{\sin \vartheta }}\;\left|{\frac {db}{d\vartheta }}\right|\ ,$

where $\vartheta$ is the angle between the incident and exit direction of the scattered particle, as shown in the figure.

The total scattering cross section can be defined (and computed) as the integral of the differential cross section over the whole solid angle:

$\sigma _{{\text{tot}}}=\oint d\Omega \;{\frac {d\sigma }{d\Omega }}$

It provides a measure of the strength of the interaction between the scattered particle and the scattering center.

When only considering a single collision between a particle beam and a sample at rest, an atom of the sample is usually taken as the scattering center. In collider experiments, both collision partners are accelerated, so either can be the scattered particle or the scattering center. When a particle is scattered by an extended body, multiple scattering centers may have to be taken into account.

Example: elastic collision of two hard spheres

The elastic collision of two hard spheres is an instructive example that demonstrates the sense of calling this quantity a cross section. $R$ and $r$ are the radii of the scattering center and scattered sphere, respectively, $b$ the impact parameter and $\vartheta$ the polar angle of the exit trajectory as above. Then the differential scattering cross section is

$\left|{\frac {d\sigma }{d\Omega }}\right|={\frac 14}(r+R)^{2}$

The total cross section is

$\sigma _{{\text{tot}}}={\frac \pi 4}\;(r+R)^{2}\ .$

So in this case the total scattering cross section is proportional to the area of the circle (with radius $r+R$ ) within which the center of mass of the incoming sphere has to arrive for it to be deflected, and outside which it passes by the stationary scattering center.

Units

The SI unit of total cross sections is the square meter, m², although smaller units are usually used in practice. The name cross-section arises because it has the dimensions of area.

When the scattered radiation is visible light, it is conventional to measure the path length in centimetres. To avoid the need for conversion factors, the scattering cross-section is expressed in cm² (1 cm² = 10⁻⁴ m²) and the number concentration in cm⁻³ (1 cm⁻³ = 10⁻⁶ m⁻³). The measurement of the scattering of visible light is known as nephelometry, and is effective for particles of 2–50 µm in diameter: as such, it is widely used in meteorology and in the measurement of atmospheric pollution.

The scattering of X-rays can also be described in terms of scattering cross-sections, in which case the square ångström, Å², is a convenient unit: 1 Å² = 10⁻²⁰ m² = 10⁴ pm².

In particle physics, where scattering processes between subatomic particles are investigated, the conventional unit is the barn, b, where 1 b = 10⁻²⁸ m² = 100 fm².^[1] Smaller prefixed units such as mb (millibarn), μb (microbarn) etc. are also widely used. The name of the unit barn originates from the fact that a scattering cross section of the order of a barn is unusually large, "big as a barn door".

The physics of scattering with a single scattering center

Classical Mechanics

In classical mechanics, the differential cross section is defined as follows: let a beam of intensity $I_{0}$ (measured in number of particles per area per time) be incident on a scattering center. In general, the angle at which a particle is scattered is a function of impact parameter. The number of scattered particles per solid angle per time (the radiant intensity), $I_{{\text{s}}}$ is therefore well defined. We define the differential cross section to be

${d\sigma \over d\Omega }={\frac {I_{{\text{s}}}}{I_{0}}}.$

Note that this quantity has units of area. Furthermore, it depends only on the geometry of the scattering center, and not on the incident flux or distance of the detector from the scattering center. The geometric interpretation is as follows: consider particles that scatter through a solid angle 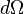$d\Omega$ and ask what values of impact parameter produced them. These impact parameters form a differential area, $d\sigma$ in space. The differential cross section is simply

${d\sigma \over d\Omega }.$

Quantum Mechanics

In quantum mechanics, the wave function of the incident particle is a plane wave with amplitude 1, that is, 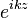$e^{{ikz}}$ . In general the scattered wave is of the form

$f(\theta ,\phi ){\frac {e^{{ikr}}}{r}}.$

We then have as the definition of differential cross section

${d\sigma \over d\Omega }=|f|^{2}.$

This has the simple interpretation of the probability of finding a scattered particle within a given solid angle.

The integral cross section is the integral of the differential cross section on the whole sphere of observation (4π steradian):

$\sigma =\int {d\sigma \over d\Omega }\,d\Omega .$

A cross section is therefore a measure of the effective surface area seen by the impinging particles, and as such is expressed in units of area. Usual units are the cm², the barn (1 b = 10⁻²⁸ m²) and the corresponding submultiples: the millibarn (1 mb = 10⁻³ b), the microbarn (1 $\mu$ b = 10⁻⁶ b), the nanobarn ( 1 nb = 10⁻⁹ b), the picobarn (1 pb = 10⁻¹² b), and the shed (1 shed = 10⁻²⁴ b). The cross section of two particles (i.e. observed when the two particles are colliding with each other) is a measure of the interaction event between the two particles. 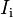The cross section is proportional to the probability that an interaction will occur; for example in a simple scattering experiment the number of particles scattered per unit of time (current of scattered particles $I_{r}$ ) depends only on the number of incident particles per unit of time (current of incident particles $I_{{\text{i}}}$ ), the characteristics of target (for example the number of particles per unit of surface N), and the type of interaction. For 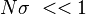$N\sigma \ <<1$ we have

$I_{{\text{r}}}=I_{{\text{i}}}N\sigma \,$

$\sigma ={{I_{{\text{r}}}} \over {I_{{\text{i}}}}}{{1} \over {N}}={{\hbox{Probability of interaction}}}\times {{1} \over {N}}$

Relation to the S matrix

If the reduced masses and momenta of the colliding system are m_i, p_i and m_f, p_f before and after the collision respectively, the differential cross section is given by

${d\sigma \over d\Omega }=(2\pi )^{4}m_{i}m_{f}{p_{f} \over p_{i}}|T_{{fi}}|^{2},$

where the on-shell T matrix is defined by

$S_{{fi}}=\delta _{{fi}}-2\pi i\delta (E_{f}-E_{i})\delta ({\mathbf {p}}_{i}-{\mathbf {p}}_{f})T_{{fi}}$

in terms of the scattering matrix S. Here, $\delta$ is the Dirac delta function. The computation of the S matrix is the main aim of the scattering theory.

Particle physics

Differential and total scattering cross sections are among the most important measurable quantities in particle physics. Instead of the solid angle, the momentum transfer is often chosed as the independent variable of differential cross sections.

Differential cross sections in inelastic scattering contain peaks indicating the creation of particles, their energy and lifetime.

The total cross section in inelastic scattering is the sum of the total cross sections of all allowed individual processes. As a consequence, total cross sections of the creation of hadrons (i.e., strongly interacting particles) receive a factor of 3 from the quarks' colour symmetry, allowing scientists to discover this symmetry.

Scattering of light on extended bodies

In the context of scattering light on extended bodies, the scattering cross-section, σ_scat, describes the likelihood of light being scattered by a macroscopic particle. In general, the scattering cross-section is different from the geometrical cross-section of a particle as it depends upon the wavelength of light and the permittivity in addition to the shape and size of the particle. The total amount of scattering in a sparse medium is determined by the product of the scattering cross-section and the number of particles present. In terms of area, the total cross-section (σ) is the sum of the cross-sections due to absorption, scattering and luminescence

$\sigma =\sigma _{{\text{A}}}+\sigma _{{\text{S}}}+\sigma _{{\text{L}}}.\$

The total cross-section is related to the absorbance of the light intensity through Beer-Lambert's law, which says absorbance is proportional to concentration: $A_{\lambda }=Cl\sigma$ , where A_λ is the absorbance at a given wavelength λ, C is the concentration as a number density, and l is the path length. The extinction or absorbance of the radiation is the logarithm (decadic or, more usually, natural) of the reciprocal of the transmittance:^[2]

$A_{\lambda }=-\log {\mathcal {T}}.\$

Relation to physical size

There is no simple relationship between the scattering cross-section and the physical size of the particles, as the scattering cross-section depends on the wavelength of radiation used. This can be seen when driving in foggy weather: the droplets of water (which form the fog) scatter red light less than they scatter the shorter wavelengths present in white light, and the red rear fog light can be distinguished more clearly than the white headlights of an approaching vehicle. That is to say that the scattering cross-section of the water droplets is smaller for red light than for light of shorter wavelengths, even though the physical size of the particles is the same.

Meteorological range

The scattering cross-section is related to the meteorological range, L_V:

$L_{{\text{V}}}={\frac {3.9}{C\sigma _{{\text{scat}}}}}.\$

The quantity C σ_scat is sometimes denoted b_scat, the scattering coefficient per unit length.^[3]

References

↑ International Bureau of Weights and Measures (2006), The International System of Units (SI) (8th ed.), pp. 127–28, ISBN 92-822-2213-6
↑ Bajpai, P.K. "2. Spectrophotometry". Biological Instrumentation and Biology. ISBN 81-219-2633-5.
↑ IUPAC, Compendium of Chemical Terminology, 2nd ed. (the "Gold Book") (1997). Online corrected version: (2006–) "Scattering cross-section, σ_scat".

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.