Scale analysis (mathematics)
Scale analysis is a powerful tool used in the mathematical sciences for the simplification of equations with many terms. First the approximate magnitude of individual terms in the equations is determined. Then some negligibly small terms may be ignored.
Example: vertical momentum in synoptic-scale meteorology
Consider for example the momentum equation of the Navier–Stokes equations in the vertical coordinate direction of the atmosphere
where R is Earth radius, Ω is frequency of rotation of the Earth, g is gravitational acceleration, φ is latitude ρ is density of air and ν is kinematic viscosity of air (we can neglect turbulence in free atmosphere).
In synoptic scale we can expect horizontal velocities about U = 101 m.s−1 and vertical about W = 10−2 m.s−1. Horizontal scale is L = 106 m and vertical scale is H = 104 m. Typical time scale is T = L/U = 105 s. Pressure differences in troposphere are ΔP = 104 Pa and density of air ρ = 100 kg·m−3. Other physical properties are approximately:
- R = 6.378 × 106 m;
- Ω = 7.292 × 10-5 rad·s−1;
- ν = 1.46 × 10−5 m2·s−1;
- g = 9.81 m·s−2.
Estimates of the different terms in equation (1) can be made using their scales:
Now we can introduce these scales and their values into equation (1):
We can see that all terms — except the first and second on the right-hand side — are negligibly small. Thus we can simplify the vertical momentum equation to the hydrostatic equilibrium equation:
See also
References
- Barenblatt, G. I. (1996). Scaling, self-similarity, and intermediate asymptotics. Cambridge University Press. ISBN 0-521-43522-6.
- Tennekes, H.; Lumley, John L. (1972). A first course in turbulence. MIT Press, Cambridge, Massachutes. ISBN 0-262-20019-8.
External links
| The Wikibook Partial Differential Equations has a page on the topic of: Scale Analysis |
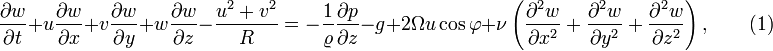
![{\begin{aligned}{{\partial w} \over {\partial t}}&\sim {\frac {W}{T}}\\[1.2ex]u{{\frac {\partial w}{\partial x}}}&\sim U{\frac {W}{L}}&\qquad v{{\frac {\partial w}{\partial y}}}&\sim U{\frac {W}{L}}&\qquad w{{\frac {\partial w}{\partial z}}}&\sim W{\frac {W}{H}}\\[1.2ex]{{\frac {u^{2}}{R}}}&\sim {\frac {U^{2}}{R}}&\qquad {{\frac {v^{2}}{R}}}&\sim {\frac {U^{2}}{R}}\\[1.2ex]{\frac {1}{\varrho }}{\frac {\partial p}{\partial z}}&\sim {\frac {1}{\varrho }}{\frac {\Delta P}{H}}&\qquad \Omega u\cos \varphi &\sim \Omega U\\[1.2ex]\nu {\frac {\partial ^{2}w}{\partial x^{2}}}&\sim \nu {\frac {W}{L^{2}}}&\qquad \nu {\frac {\partial ^{2}w}{\partial y^{2}}}&\sim \nu {\frac {W}{L^{2}}}&\qquad \nu {\frac {\partial ^{2}w}{\partial z^{2}}}&\sim \nu {\frac {W}{H^{2}}}\end{aligned}}](/2014-wikipedia_en_all_02_2014/I/media/4/a/6/9/4a696736fd410ef987a83433039876bc.png)


