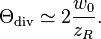Rayleigh length

From Wikipedia, the free encyclopedia
Gaussian beam width w(z) as a function of the axial distance z. w_{0}: beam waist; b: confocal parameter; z_{{\mathrm {R}}}: Rayleigh length; 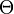\Theta : total angular spread

In optics and especially laser science, the Rayleigh length or Rayleigh range is the distance along the propagation direction of a beam from the waist to the place where the area of the cross section is doubled.[1] A related parameter is the confocal parameter, b, which is twice the Rayleigh length.[2] The Rayleigh length is particularly important when beams are modeled as Gaussian beams.

Explanation

For more details on this topic, see Gaussian beam.

For a Gaussian beam propagating in free space along the {\hat {z}} axis, the Rayleigh length is given by [2]

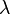z_{{\mathrm {R}}}={\frac {\pi w_{0}^{2}}{\lambda }},

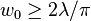where \lambda is the wavelength and w_{0} is the beam waist, the radial size of the beam at its narrowest point. This equation and those that follow assume that the waist is not extraordinarily small; w_{0}\geq 2\lambda /\pi .[3]

The radius of the beam at a distance z from the waist is [4]

w(z)=w_{0}\,{\sqrt {1+{\left({\frac {z}{z_{{\mathrm {R}}}}}\right)}^{2}}}.

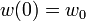The minimum value of w(z) occurs at w(0)=w_{0}, by definition. At distance z_{{\mathrm {R}}} from the beam waist, the beam radius is increased by a factor {\sqrt {2}} and the cross sectional area by 2.

Related quantities

The total angular spread of a Gaussian beam in radians is related to the Rayleigh length by[1]

\Theta _{{{\mathrm {div}}}}\simeq 2{\frac {w_{0}}{z_{R}}}.

The diameter of the beam at its waist (focus spot size) is given by

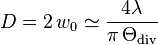D=2\,w_{0}\simeq {\frac {4\lambda }{\pi \,\Theta _{{{\mathrm {div}}}}}}.

These equations are valid within the limits of the paraxial approximation. For beams with much larger divergence the Gaussian beam model is no longer accurate and a physical optics analysis is required.

See also

References

  1. ↑ 1.0 1.1 Siegman, A. E. (1986). Lasers. University Science Books. pp. 664–669. ISBN 0-935702-11-3. 
  2. ↑ 2.0 2.1 Damask, Jay N. (2004). Polarization Optics in Telecommunications. Springer. pp. 221–223. ISBN 0-387-22493-9. 
  3. ↑ Siegman (1986) p. 630.
  4. ↑ Meschede, Dieter (2007). Optics, Light and Lasers: The Practical Approach to Modern Aspects of Photonics and Laser Physics. Wiley-VCH. pp. 46–48. ISBN 3-527-40628-X. 
This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.