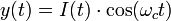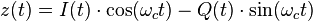Quadrature modulation

From Wikipedia, the free encyclopedia

Quadrature modulation is the general technique of modulating two carriers.

Examples include Quadrature amplitude modulation, Phase-shift keying, and Minimum-shift keying.

Constellation diagrams are used to examine the modulation in the 2-D signal space.

Explanation

Sending a signal by amplitude modulation consists of sending the function

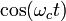$y(t)=I(t)\cdot \cos(\omega _{c}t)$

where $I(t)$ is the signal to encode and $\cos(\omega _{c}t)$ is the carrier wave, $\omega _{c}$ is the carrier frequency – one is changing the amplitude of a carrier wave to encode the signal, hence amplitude modulation.

In general one could also change the phase of the carrier wave, as in phase modulation – there is a dimension of phase that is not being used. In fact, one can encode another signal that is 90° out of phase by using a sine wave, as in:

$z(t)=I(t)\cdot \cos(\omega _{c}t)-Q(t)\cdot \sin(\omega _{c}t)$

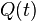this 90° (the angle of a rectangle, or a 1/4 turn) is why it is called "quadrature" modulation, and the symbols $I(t)$ and $Q(t)$ indicate the "in-phase" signal and "quadrature" signal.

In terms of Euler's formula, 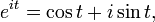$e^{{it}}=\cos t+i\sin t,$ amplitude modulation encodes a 1-dimensional real signal, while quadrature modulation encodes a 2-dimensional complex signal. This viewpoint, that a wave of a given frequency can encode 2 dimensions of data, is elaborated in Fourier analysis, and is the principle that quadrature modulation exploits.

Clocking

The added channel capacity is not costless, however.

An amplitude-modulated signal is self-clocking – it has zero-crossings at a regular frequency as a clock pulse. A quadrature-modulated signal, by contrast, has no such pulse, and thus sender and receiver must share a clock or otherwise send a clock signal – if the clocks drift by phase φ, which corresponds to rotation by φ in the 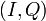$(I,Q)$ plane, then the I and Q signal bleed into each other, yielding crosstalk. In this context, the clock signal is called a "phase reference" – in NTSC, which uses quadrature amplitude modulation, this is conveyed by the color burst, a synchronization signal.

By contrast, in polar modulation, clock drift simply degrades the phase-modulated signal.

Polar modulation

Main article: Polar modulation

Quadrature modulates two signals by changing the in-phase and quadrature phase components, corresponding to Cartesian coordinates. By contrast, one can instead consider this to be changing the amplitude and phase of a wave, which corresponds to polar coordinates. The corresponding modulation is called polar modulation, and was developed earlier, in the 1874 quadruplex telegraph by Thomas Edison.

Quadrature modulation

Explanation

Clocking

Polar modulation

See also