Pulse wave velocity

From Wikipedia, the free encyclopedia

Pulse wave velocity (PWV) is a measure of arterial stiffness.[1][2] It is easy to measure invasively and non-invasively in humans, is highly reproducible,[3] has a strong correlation with cardiovascular events and all-cause mortality,[4][5][6][7] and was recognized by the European Society of Hypertension as integral to the diagnosis and treatment of hypertension.[8]

Relationship between arterial stiffness and pulse wave velocity

The study of the basic scientific principles of the velocity of the pulse wave through the arterial tree dates back to 1808 with the work of Thomas Young.[9] The relationship between pulse wave velocity (PWV) and arterial wall stiffness can be calculated from first principles from Newton's second law of motion;

F=ma

Using some simplifying assumptions, the Moens–Korteweg equation can be derived,[10][11] an equation that directly relates PWV and artery wall stiffness.

PWV={\sqrt {{\dfrac {E_{{inc}}\cdot h}{2r\rho }}}}

The Moens-Korteweg equation states that PWV is proportional to the square root of the incremental elastic modulus of the vessel wall given constant ratio of wall thickness h to vessel radius r[11][12] under the assumptions used to derive the equation, these assumptions being:

  1. there is no, or insignificant, change in vessel area.
  2. there is no, or insignificant, change in wall thickness.
  3. that dv(dr^{{-1}})dx\cdot dt is small to the point of insignificance.

Measuring pulse wave velocity

Using the velocity of the forward traveling wave

PWV, by definition, is the distance traveled (\Delta x) by the wave divided by the time (\Delta t) for the wave to travel that distance:

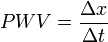
PWV={\dfrac {\Delta x}{\Delta t}}

This holds true for a system with zero wave reflections. The transmission of the arterial pressure pulse does not give the true PWV as it is a sum of vectors of the incident and reflected waves. Therefore, appropriate pressure and flow measurements must be made to estimate the characteristic impedance and to calculate the incident, or the reflected pressure wave at two separate locations a known distance apart (although there might be come conceptual problems with the term “wave reflection” in the arterial system).

Using two simultaneously measured pressure waves

An alternate method of measuring PWV utilizes the feature of the arterial waveform that during late diastole and early systole, there is no, or minimal, interference of the incident pressure wave by the reflected pressure wave.[13] With this assumption, PWV can be measured between two sites a known distance apart using the pressure `foot' of the waveform to calculate the transit time. Exactly locating the pressure waveform foot can be subjective and less than accurate.[11] The advantage of foot-to-foot PWV measurement is the simplicity of measurement, requiring only two pressure wave forms recorded with invasive catheters, or mechanical tonometers or pulse detection devices applied non-invasively to the pulse across the skin, where the site of the two measurements are a known distance apart.[14]

Using pressure and flow

Bramwell & Hill[15] cited the Moens-Kortweg equation and proposed a series of substitutions relevant to observable haemodynamic measures. Quoting directly, these substitutions were:

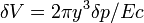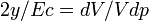"A small rise \delta p in pressure may be shown to cause a small increase, \delta y=y^{2}\delta p/Ec, in the radius y of the artery, or a small increase, \delta V=2\pi y^{{3}}\delta p/Ec, in its own volume V per unit length. Hence 2y/Ec=dV/Vdp"

where c represents the wall thickness (usually defined as h) and y the vessel radius (usually defined as r). Substituting these observations into the Moens-Korteweg equation gives the Bramwell-Hill equation with wave speed in terms of 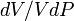dV/VdP. This provides an alternate method of measuring PWV, where pressure can be measured, and flow and arterial dimension measured through techniques such as A or M-mode ultrasound or Doppler measurement of flow.

PWV={\sqrt {{\dfrac {dP\cdot V}{\rho \cdot dV}}}}

A similarity between the Moens-Kortweg equation and Newton's equation for the wave speed in a material is evident and both the Moens-Kortweg and Bramwell-Hill equations can be derived from Newton's equation for wave speed using the substitution of the equation of the bulk modulus in terms of volumetric strain.

Using characteristic impedance

The Waterhammer equation[16][17] gives another alternate expression of PWV. The equation directly relates characteristic impedance (Z_{{c}}) to PWV through the ratio of pressure (P) and linear flow velocity (v) in the absence of wave reflection. Subsequently, an estimate of characteristic impedance through pressure and flow measurement provides a measure of PWV, which is proportional to arterial stiffness.

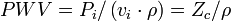
PWV=P_{{i}}/\left(v_{{i}}\cdot \rho \right)=Z_{{c}}/\rho

Nomenclature

  • \rho density (of blood)
  • h vessel wall thickness
  • E_{{inc}} incremental modulus of stiffness
  • P arterial blood pressure
  • PWVpulse wave velocity
  • r vessel radius
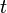  • t time
  • V blood volume
  • v velocity
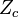  • Z_{c} characteristic impedance

References

  1. ↑ Wilkinson IB, Cockcroft JR, Webb DJ (1998). "Pulse wave analysis and arterial stiffness". J. Cardiovasc. Pharmacol. 32 (Suppl 3): S33–7. PMID 9883745. 
  2. ↑ Nichols WW (January 2005). "Clinical measurement of arterial stiffness obtained from noninvasive pressure waveforms". Am. J. Hypertens. 18 (1 Pt 2): 3S–10S. doi:10.1016/j.amjhyper.2004.10.009. PMID 15683725. 
  3. ↑ Wilkinson IB, Fuchs SA, Jansen IM, et al. (December 1998). "Reproducibility of pulse wave velocity and augmentation index measured by pulse wave analysis". J. Hypertens. 16 (12 Pt 2): 2079–84. doi:10.1097/00004872-199816121-00033. PMID 9886900. 
  4. ↑ Blacher J, Asmar R, Djane S, London GM, Safar ME (May 1999). "Aortic pulse wave velocity as a marker of cardiovascular risk in hypertensive patients". Hypertension 33 (5): 1111–7. doi:10.1161/01.HYP.33.5.1111. PMID 10334796. 
  5. ↑ Blacher J, Guerin AP, Pannier B, Marchais SJ, Safar ME, London GM (May 1999). "Impact of aortic stiffness on survival in end-stage renal disease". Circulation 99 (18): 2434–9. doi:10.1161/01.CIR.99.18.2434. PMID 10318666. 
  6. ↑ Cruickshank K, Riste L, Anderson SG, Wright JS, Dunn G, Gosling RG (October 2002). "Aortic pulse-wave velocity and its relationship to mortality in diabetes and glucose intolerance: an integrated index of vascular function?". Circulation 106 (16): 2085–90. doi:10.1161/01.CIR.0000033824.02722.F7. PMID 12379578. 
  7. ↑ Laurent S, Boutouyrie P, Asmar R, et al. (May 2001). "Aortic stiffness is an independent predictor of all-cause and cardiovascular mortality in hypertensive patients". Hypertension 37 (5): 1236–41. doi:10.1161/01.HYP.37.5.1236. PMID 11358934. 
  8. ↑ Mancia G, De Backer G, Dominiczak A, et al. (June 2007). "2007 Guidelines for the Management of Arterial Hypertension: The Task Force for the Management of Arterial Hypertension of the European Society of Hypertension (ESH) and of the European Society of Cardiology (ESC)". J. Hypertens. 25 (6): 1105–87. doi:10.1097/HJH.0b013e3281fc975a. PMID 17563527. 
  9. ↑ Young T (1809). "The Croonian Lecture: On the functions of the heart and arteries". Philosophical Transactions of the Royal Society of London 99: 1–31. doi:10.1098/rstl.1809.0001. 
  10. ↑ Butlin M (2007). "Structural and function effects on large artery stiffness: an in-vivo experimental investigation". Graduate School of Biomedical Engineering, University of New South Wales. 
  11. ↑ 11.0 11.1 11.2 Milnor, William R. (1982). Hemodynamics. Baltimore: Williams & Wilkins. ISBN 0-683-06050-3. 
  12. ↑ McDonald, Donald A.; Nichols, Wilmer W.; O'Rourke, Michael J.; Hartley, Craig (1998). McDonald's Blood Flow in Arteries, Theoretical, experimental and clinical principles (4th ed.). London: Arnold. ISBN 0-340-64614-4. 
  13. ↑ Bramwell JC, Hill AV (1922). "Velocity transmission of the pulse wave and elasticity of arteries". Lancet 199 (5149): 891–2. doi:10.1016/S0140-6736(00)95580-6. 
  14. ↑ Boutouyrie P, Briet M, Collin C, Vermeersch S, Pannier B (February 2009). "Assessment of pulse wave velocity". Artery Research 3 (1): 3–8. doi:10.1016/j.artres.2008.11.002. 
  15. ↑ Bramwell JC, Hill AV (1922). "The velocity of the pulse wave in man". Proceedings of the Royal Society of London. Series B 93 (652): 298–306. doi:10.1098/rspb.1922.0022. JSTOR 81045. 
  16. ↑ O'Rourke MF (April 1982). "Vascular impedance in studies of arterial and cardiac function". Physiol. Rev. 62 (2): 570–623. PMID 6461866. 
  17. ↑ Murgo JP, Westerhof N, Giolma JP, Altobelli SA (July 1980). "Aortic input impedance in normal man: relationship to pressure wave forms". Circulation 62 (1): 105–16. doi:10.1161/01.CIR.62.1.105. PMID 7379273. 
This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.