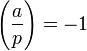Proth's theorem

From Wikipedia, the free encyclopedia

In number theory, Proth's theorem is a primality test for Proth numbers.

It states that if p is a Proth number, of the form k2ⁿ + 1 with k odd and k < 2ⁿ, then if for some integer a,

$a^{{(p-1)/2}}\equiv -1\mod {p}\,\!$

then p is prime (called a Proth prime). This is a practical test because if p is prime, any chosen a has about a 50 percent chance of working.

If a is a quadratic nonresidue modulo p then the converse is also true, and the test is conclusive. Such an a may be found by iterating a over small primes and computing the Jacobi symbol until:

$\left({\frac {a}{p}}\right)=-1$

Numerical examples

Examples of the theorem include:

for p = 3, 2¹ + 1 = 3 is divisible by 3, so 3 is prime.
for p = 5, 3² + 1 = 10 is divisible by 5, so 5 is prime.
for p = 13, 5⁶ + 1 = 15626 is divisible by 13, so 13 is prime.
for p = 9, which is not prime, there is no a such that a⁴ + 1 is divisible by 9.

The first Proth primes are (sequence A080076 in OEIS):

3, 5, 13, 17, 41, 97, 113, 193, 241, 257, 353, 449, 577, 641, 673, 769, 929, 1153 ….

As of 2009, the largest known Proth prime is 19249 · 2^13018586 + 1, found by Seventeen or Bust. It has 3,918,990 digits and is the largest known prime which is not a Mersenne prime. ^[1]

History

François Proth (1852–1879) published the theorem around 1878.

References

↑ http://primes.utm.edu/top20/page.php?id=3

External links

Weisstein, Eric W., "Proth's Theorem", MathWorld.

v t e Number-theoretic algorithms

Primality tests	AKS test APR test Baillie–PSW ECPP test Elliptic curve Pocklington Fermat Lucas Lucas–Lehmer Lucas–Lehmer–Riesel Proth's theorem Pépin's Quadratic Frobenius test Solovay–Strassen Miller–Rabin Trial division

Prime-generating	Sieve of Atkin Sieve of Eratosthenes Sieve of Sundaram Wheel factorization

Integer factorization	Continued fraction (CFRAC) Dixon's Lenstra elliptic curve (ECM) Euler's Pollard's rho p − 1 p + 1 Quadratic sieve (QS) General number field sieve (GNFS) Special number field sieve (SNFS) Rational sieve Fermat's Shanks' square forms Trial division Shor's

Multiplication	Ancient Egyptian Karatsuba Toom–Cook Schönhage–Strassen Fürer's

Discrete logarithm	Baby-step giant-step Pollard rho Pollard kangaroo Pohlig–Hellman Index calculus Function field sieve

Greatest common divisor	Binary Euclidean Extended Euclidean Lehmer's

Modular square root	Cipolla Pocklington's Tonelli–Shanks

Other algorithms	Chakravala Cornacchia Integer relation Integer square root Modular exponentiation Schoof's

Italics indicate that algorithm is for numbers of special forms Smallcaps indicate a deterministic algorithm

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.

Proth's theorem

Numerical examples

History

See also

References

External links