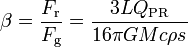Poynting–Robertson effect

From Wikipedia, the free encyclopedia

The Poynting–Robertson effect, also known as Poynting–Robertson drag, named after John Henry Poynting and Howard Percy Robertson, is a process by which solar radiation causes a dust grain in the Solar System to spiral slowly into the Sun. The drag is essentially a component of radiation pressure tangential to the grain's motion. Poynting gave a description of the effect in 1903 based on the "luminiferous aether" theory, which was superseded by the theories of relativity in 1905–1915. In 1937 Robertson described the effect in terms of general relativity.

Explanation

The effect can be understood in two ways, depending on the reference frame chosen.

Radiation from the Sun (S) and thermal radiation from a particle seen (a) from an observer moving with the particle and (b) from an observer at rest with respect to the Sun.

From the perspective of the grain of dust circling the Sun (panel (a) of the figure), the Sun's radiation appears to be coming from a slightly forward direction (aberration of light). Therefore the absorption of this radiation leads to a force with a component against the direction of movement. The angle of aberration is extremely small since the radiation is moving at the speed of light while the dust grain is moving many orders of magnitude slower than that.

From the perspective of the Solar System as a whole (panel (b) of the figure), the dust grain absorbs sunlight entirely in a radial direction, thus the grain's angular momentum is not affected by it. But the re-emission of photons, which is isotropic in the frame of the grain (a), is no longer isotropic in the frame of the Solar System (b). This anisotropic emission causes the photons to carry away angular momentum from the dust grain.

The Poynting–Robertson drag can be understood as an effective force opposite the direction of the dust grain's orbital motion, leading to a drop in the grain's angular momentum. It should be mentioned that while the dust grain thus spirals slowly into the Sun, its orbital speed increases continuously.

The Poynting–Robertson force is equal to:

F_{{{\rm {PR}}}}={\frac {v}{c^{2}}}W={\frac {r^{2}L_{{{\rm {s}}}}}{4c^{2}}}{\sqrt {{\frac {GM_{{{\rm {s}}}}}{R^{5}}}}}

where v is the grain's velocity, c is the speed of light, W is the power of the incoming radiation, r the grain's radius, G is the universal gravitational constant, Ms the Sun's mass, Ls is the solar luminosity and R the grain's orbital radius.

Since the gravitational force goes as the cube of the object's radius (being a function of its volume) whilst the power it receives and radiates goes as the square of that same radius (being a function of its surface), the Poynting–Robertson effect is more pronounced for smaller objects. Also, since the Sun's gravity varies as 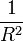{\frac {1}{R^{2}}} whereas the Poynting–Robertson force varies as 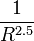{\frac {1}{R^{{2.5}}}}, the Poynting–Robertson effect also gets relatively stronger as the object approaches the Sun, which tends to reduce the eccentricity of the object's orbit in addition to dragging it in.

Rocky dust particles sized a few micrometers need a few thousand years to get from 1 AU distance to distances where they evaporate.

For particles much smaller than this, radiation pressure, which makes them spiral outwards from the Sun, is stronger than the Poynting–Robertson effect that makes them spiral inward. For rocky particles about half a µm in diameter, the radiation pressure equals gravity, and they will be always blown out of the Solar System even though the Poynting–Robertson effect still affects them.[1] Particles of intermediate size will either spiral inwards or outwards depending on their size and their initial velocity vector.

In addition, as the size of the particle increases, the surface temperature is no longer approximately constant, and the radiation pressure is no longer isotropic in the particle's reference frame. If the particle rotates slowly, the radiation pressure may contribute to the change in angular momentum, either positively or negatively.

Robertson considered dust motion in a beam of radiation emanating from a point source. Guess also considered the problem but for a spherical source of radiation and found that for particles far from the source the resultant forces are in agreement with those concluded by Robertson.[2]

The dimensionless dust parameter \beta is the ratio of the force due to radiation pressure to the force of gravity on the particle:

\beta ={F_{{{\rm {r}}}} \over F_{{{\rm {g}}}}}={3LQ_{{{\rm {PR}}}} \over {16\pi GMc\rho s}}

where Q_{{{\rm {PR}}}} is the Mie scattering coefficient, and \rho is the density and s is the size (the radius) of the dust grain.[3]

The Equations of Motion for the dust grain are expressed by

m{\operatorname {d^{2}}{\vec {x}} \over \operatorname {d}t^{2}}=-GMm{\Big (}1-\beta {\Big )}{{\vec {x}} \over r^{3}}+GMm\beta {\Bigg \{}{-{{{\vec {x}}\cdot {\vec {v}}} \over {cr}}{{\vec {x}} \over r^{3}}-{{\vec {v}} \over {cr^{2}}}+{R_{{{\rm {s}}}}^{2} \over {cr^{4}}}{\Big (}{\vec {\omega }}\times {\vec {x}}{\Big )}}{\Bigg \}}

where R_{{{\rm {s}}}} is the stellar radius.[4]

Notes

  1. ↑ http://www.britannica.com/eb/article-9126477
  2. ↑ Guess, A. W. (1962). "Poynting-Robertson Effect for a Spherical Source of Radiation". Astrophysical Journal 135: 855–866. Bibcode:1962ApJ...135..855G. doi:10.1086/147329. 
  3. ↑ Burns; Lamy; Soter (1979). "Radiation Forces on Small Particles in the Solar System". Icarus 40 (1): 1–48. Bibcode:1979Icar...40....1B. doi:10.1016/0019-1035(79)90050-2. 
  4. ↑ Kressel, J. H. (1996). "Dust Dynamics in Nascent Protoplanetary Disks". Masters Thesis (Old Dominion University). 

See also

References

  • Poynting, J. H. (November 1903). "Radiation in the solar system: its Effect on Temperature and its Pressure on Small Bodies". Monthly Notices of the Royal Astronomical Society (Royal Astronomical Society) 64 (Appendix): 1–5. Bibcode:1903MNRAS..64A...1P.  (Abstract of Philosophical Transactions paper)
  • Robertson, H. P. (April 1937). "Dynamical effects of radiation in the solar system". Monthly Notices of the Royal Astronomical Society (Royal Astronomical Society) 97: 423–438. Bibcode:1937MNRAS..97..423R. 
This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.