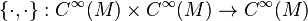Poisson manifold
In mathematics, a Poisson manifold is a smooth manifold  equipped with a bilinear map
equipped with a bilinear map 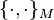 (called a Poisson bracket) on the algebra
(called a Poisson bracket) on the algebra  of smooth functions on
of smooth functions on  such that
such that 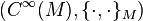 is a Poisson algebra. One usually denotes a Poisson manifold by the ordered pair
is a Poisson algebra. One usually denotes a Poisson manifold by the ordered pair  . Since their introduction by André Lichnerowicz in 1977,[1] the subjects of Poisson geometry and the cohomology of Poisson manifolds have developed into a wide field of research, which includes modern-day non-commutative geometry.
. Since their introduction by André Lichnerowicz in 1977,[1] the subjects of Poisson geometry and the cohomology of Poisson manifolds have developed into a wide field of research, which includes modern-day non-commutative geometry.
It is a fact that every symplectic manifold is a Poisson manifold but not vice-versa. This will be explained in Section 2.
Definition
A Poisson bracket (or Poisson structure) on a smooth manifold  is a bilinear map
is a bilinear map
that satisfies the following three properties:
- It is skew-symmetric:
 .
. - It obeys the Jacobi Identity:
 .
. - It obeys Leibniz's Rule with respect to the first argument:
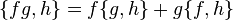 .
.
By skew-symmetry, the Poisson bracket automatically satisfies Leibniz's Rule with respect to the second argument. The last property basically states that the map 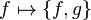 is a derivation on
is a derivation on  for any fixed
for any fixed 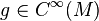 . Every derivation
. Every derivation  on
on  can be written as a directional derivative
can be written as a directional derivative ={({\mathrm {d}}f)_{{x}}}((X_{{\delta }})_{{x}})](/2014-wikipedia_en_all_02_2014/I/media/9/e/9/e/9e9ee342c5e411cf3c176853cc486e37.png) , where
, where  , for some vector field
, for some vector field 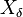 . It follows that for
. It follows that for 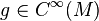 , we obtain a vector field
, we obtain a vector field 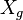 such that
such that 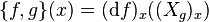 , where
, where  (written more briefly,
(written more briefly, 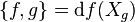 ). The vector field
). The vector field 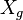 is called the Hamiltonian vector field corresponding to
is called the Hamiltonian vector field corresponding to 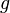 . Notice that
. Notice that
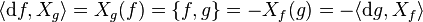 ,
,
where  is the pairing between the cotangent and tangent bundles of
is the pairing between the cotangent and tangent bundles of  . Therefore,
. Therefore, 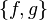 depends only on the differentials
depends only on the differentials  and
and  . Any Poisson bracket yields a map from the cotangent bundle to the tangent bundle that sends
. Any Poisson bracket yields a map from the cotangent bundle to the tangent bundle that sends  to
to 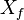 .
.
The Poisson Bivector
Given a Poisson manifold  , the pairing between the cotangent and tangent bundles yields a bivector field
, the pairing between the cotangent and tangent bundles yields a bivector field  on
on  , called the Poisson bivector field. The Poisson bivector field is a contravariant skew-symmetric 2-tensor field
, called the Poisson bivector field. The Poisson bivector field is a contravariant skew-symmetric 2-tensor field  that satisfies the following:
that satisfies the following:
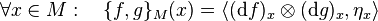 .
.
Conversely, given a smooth bivector field  on
on  , we can use the formula above to define a skew-symmetric bracket
, we can use the formula above to define a skew-symmetric bracket 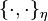 that obeys Leibniz's rule with respect to each argument. However, we cannot claim that
that obeys Leibniz's rule with respect to each argument. However, we cannot claim that 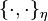 is a Poisson bracket because the Jacobi Identity may not hold (in this case, we call
is a Poisson bracket because the Jacobi Identity may not hold (in this case, we call 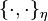 an almost-Poisson structure). Indeed,
an almost-Poisson structure). Indeed, 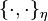 is a Poisson bracket if and only if the Schouten–Nijenhuis bracket
is a Poisson bracket if and only if the Schouten–Nijenhuis bracket ![[\eta ,\eta ]](/2014-wikipedia_en_all_02_2014/I/media/8/8/8/d/888d4c4e52240166f97c1b3e97cead35.png) equals zero.
equals zero.
In terms of local coordinates, the bivector field at a point 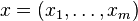 can be expressed as
can be expressed as
 ,
,
so that
 .
.
For a symplectic manifold  , we can define a bivector field
, we can define a bivector field  on
on  using the pairing between the cotangent and tangent bundles given by the symplectic form
using the pairing between the cotangent and tangent bundles given by the symplectic form 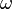 . This pairing is well-defined because
. This pairing is well-defined because 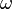 is nondegenerate. Hence, the difference between a symplectic manifold and a Poisson manifold is that the symplectic form is regular (of full rank) everywhere but the Poisson bivector field need not have full rank everywhere. When the Poisson bivector field is zero everywhere, we call it the trivial Poisson structure.
is nondegenerate. Hence, the difference between a symplectic manifold and a Poisson manifold is that the symplectic form is regular (of full rank) everywhere but the Poisson bivector field need not have full rank everywhere. When the Poisson bivector field is zero everywhere, we call it the trivial Poisson structure.
Poisson Maps
A Poisson map from a Poisson manifold  to another Poisson manifold
to another Poisson manifold 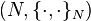 is defined to be a smooth map
is defined to be a smooth map  that respects the Poisson structures in the following sense:
that respects the Poisson structures in the following sense:
 .
.
A Poisson map may be viewed as a morphism in the category of Poisson manifolds.
The Product of Poisson Manifolds
Given two Poisson manifolds  and
and 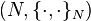 , a Poisson bracket may be defined on the product manifold
, a Poisson bracket may be defined on the product manifold  . Letting
. Letting 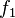 and
and 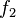 be two smooth functions defined on
be two smooth functions defined on  , one can define a new Poisson bracket
, one can define a new Poisson bracket 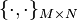 in terms of
in terms of 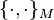 and
and  as follows:
as follows:
 ,
,
where  and
and 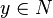 are to be held constant. In other words, if
are to be held constant. In other words, if
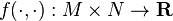 ,
,
then both
and
are implied.
The Symplectic Leaves of a Poisson Structure
A Poisson manifold  can be split into a collection of symplectic leaves. This splitting arises from the foliation of disjoint regions of
can be split into a collection of symplectic leaves. This splitting arises from the foliation of disjoint regions of  where the Poisson bivector field has constant rank. Each leaf of the foliation is thus an even-dimensional sub-manifold of
where the Poisson bivector field has constant rank. Each leaf of the foliation is thus an even-dimensional sub-manifold of  that is itself a symplectic manifold. Distinct symplectic leaves may have different dimensions. Two points lie in the same leaf if and only if they are joined by a piecewise-smooth curve where each piece is the integral curve of a Hamiltonian vector field. The relation "piecewise-connected by integral curves of Hamiltonian fields" is an equivalence relation on
that is itself a symplectic manifold. Distinct symplectic leaves may have different dimensions. Two points lie in the same leaf if and only if they are joined by a piecewise-smooth curve where each piece is the integral curve of a Hamiltonian vector field. The relation "piecewise-connected by integral curves of Hamiltonian fields" is an equivalence relation on  , and the equivalence classes of this equivalence relation are the symplectic leaves.
, and the equivalence classes of this equivalence relation are the symplectic leaves.
Example (Lie-Poisson Manifold)
If  is a finite-dimensional Lie algebra and
is a finite-dimensional Lie algebra and 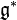 is its dual vector space, then the Lie bracket induces a Poisson structure on
is its dual vector space, then the Lie bracket induces a Poisson structure on 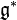 .
.
More precisely, we identify the cotangent bundle of the manifold 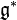 , i.e., the dual of
, i.e., the dual of 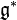 with the given Lie algebra
with the given Lie algebra  . Then for two functions
. Then for two functions 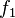 and
and 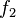 on
on 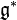 , and a point
, and a point 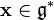 , we may define
, we may define
![\{f_{1},f_{2}\}({\mathbf {x}}):=\langle \left[({\mathrm {d}}f_{{1}})_{{{\mathbf {x}}}},({\mathrm {d}}f_{{2}})_{{{\mathbf {x}}}}\right],{\mathbf {x}}\rangle](/2014-wikipedia_en_all_02_2014/I/media/3/e/f/2/3ef227cf65b9032a95385b9e79b9f00d.png) ,
,
where the Lie bracket ![[\cdot ,\cdot ]](/2014-wikipedia_en_all_02_2014/I/media/3/5/8/7/3587c5df5edf1176ed7afc1f20f5d8a9.png) is computed in
is computed in  through the isomorphism:
through the isomorphism:
 .
.
If  are local coordinates on
are local coordinates on  , then the Poisson bivector field is given by
, then the Poisson bivector field is given by
 ,
,
where the  are the structure constants of
are the structure constants of  .
.
The symplectic leaves of this Lie-Poisson manifold are the co-adjoint orbits of the Lie algebra used for the orbit method.
Complex Structure
A complex Poisson manifold is a Poisson manifold with a complex or almost complex structure  such that the complex structure preserves the bivector:
such that the complex structure preserves the bivector:
 .
.
The symplectic leaves of a complex Poisson manifold are pseudo-Kähler manifolds.
See also
- Nambu-Poisson manifold
- Poisson-Lie group
- Poisson supermanifold
Notes
- ↑ Lichnerowicz, A. (1977). "Les variétés de Poisson et leurs algèbres de Lie associées". J. Diff. Geom. 12 (2): 253–300. MR 0501133.
References
- Lichnerowicz, A. (1977). "Les variétés de Poisson et leurs algèbres de Lie associées". J. Diff. Geom. 12 (2): 253–300. MR 0501133.
- Kirillov, A. A. (1976). "Local Lie algebras". Russ. Math. Surv. 31 (4): 55–75. doi:10.1070/RM1976v031n04ABEH001556.
- Guillemin, V.; Sternberg, S. (1984). Symplectic Techniques in Physics. New York: Cambridge Univ. Press. ISBN 0-521-24866-3.
- Libermann, P.; Marle, C.-M. (1987). Symplectic geometry and analytical mechanics. Dordrecht: Reidel. ISBN 90-277-2438-5.
- Bhaskara, K. H.; Viswanath, K. (1988). Poisson algebras and Poisson manifolds. Longman. ISBN 0-582-01989-3.
- Vaisman, I. (1994). Lectures on the Geometry of Poisson Manifolds. Birkhäuser. See also the review by Ping Xu in the Bulletin of the AMS.
- Weinstein, A. (1983). "The local structure of Poisson manifolds". J. Diff. Geom. 18 (3): 523–557. MR 834280. Errata and addenda J. Diff. Geom. 22 (1985), 255.