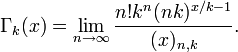Pochhammer k-symbol

From Wikipedia, the free encyclopedia

In the mathematical theory of special functions, the Pochhammer k-symbol and the k-gamma function, introduced by Rafael Díaz and Eddy Pariguan,^[1] are generalizations of the Pochhammer symbol and gamma function. They differ from the Pochhammer symbol and gamma function in that they can be related to a general arithmetic progression in the same manner as those are related to the sequence of consecutive integers.

The Pochhammer k-symbol (x)_n,k is defined as

$(x)_{{n,k}}=x(x+k)(x+2k)\cdots (x+(n-1)k),\,$

and the k-gamma function Γ_k, with k > 0, is defined as

$\Gamma _{k}(x)=\lim _{{n\to \infty }}{\frac {n!k^{n}(nk)^{{x/k-1}}}{(x)_{{n,k}}}}.$

When k = 1 the standard Pochhammer symbol and gamma function are obtained.

Díaz and Pariguan use these definitions to demonstrate a number of properties of the hypergeometric function. Although Díaz and Pariguan restrict these symbols to k > 0, the Pochhammer k-symbol as they define it is well-defined for all real k, and for negative k gives the falling factorial, while for k = 0 it reduces to the power xⁿ.

The Díaz and Pariguan paper does not address the many analogies between the Pochhammer k-symbol and the power function, such as the fact that the binomial theorem can be extended to Pochhammer k-symbols. It is true, however, that many equations involving the power function xⁿ continue to hold when xⁿ is replaced by (x)_n,k.

References

↑ Díaz, Rafael; Eddy Pariguan (2005). "On hypergeometric functions and k-Pochhammer symbol". arXiv:math/0405596 [math.CA].

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.