Petzval field curvature

From Wikipedia, the free encyclopedia
Optical aberration
Distortion

Spherical aberration
Coma
Astigmatism
Petzval field curvature
Chromatic aberration
Defocus
Tilt

Not to be confused with flat-field correction, which refers to brightness uniformity.
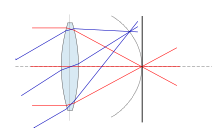
Field curvature: the image "plane" (the arc) deviates from a flat surface (the vertical line).

Petzval field curvature, named for Joseph Petzval,[1] describes the optical aberration in which a flat object normal to the optical axis (or a non-flat object past the hyperfocal distance) cannot be brought into focus on a flat image plane.[citation needed]

Analysis

The image-sensor array of the Kepler space observatory is curved to compensate for the telescope's Petzval curvature.

Consider an "ideal" single-element lens system for which all planar wave fronts are focused to a point at distance f from the lens. Placing this lens the distance f from a flat image sensor, image points near the optical axis will be in perfect focus, but rays off axis will come into focus before the image sensor, dropping off by the cosine of the angle they make with the optical axis. This is less of a problem when the imaging surface is spherical, as in the human eye.[citation needed]

Most current photographic lenses are designed to minimize field curvature, and so effectively have a focal length that increases with ray angle. However, film cameras could bend their image planes to compensate, particularly when the lens is fixed and known. This also includes plate film, which could still be bent slightly. Digital sensors generally cannot be bent, although large mosaics of sensors (necessary anyway due to limited chip sizes) can be shaped to simulate a bend over larger scales.[citation needed]

The Petzval field curvature is equal to the Petzval sum over an optical system,

\sum _{i}{\frac {n_{{i+1}}-n_{i}}{r_{i}n_{{i+1}}n_{i}}},

where r_{i} is the radius of the ith surface and the ns are the indices of refraction on the first and second side of the surface.[2]

See also

References

  1. ↑ Riedl, Max J. (2001). Optical Design Fundamentals for Infrared Systems. SPIE Press. pp. 40–. ISBN 9780819440518. Retrieved 3 November 2012. 
  2. ↑ Kingslake, Rudolf (1989). A History of the Photographic Lens. Academic Press. pp. 4–. ISBN 9780124086401. Retrieved 3 November 2012. 

External links


This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.