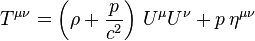Perfect fluid

From Wikipedia, the free encyclopedia
The stress–energy tensor of a perfect fluid contains only the diagonal components.

In physics, a perfect fluid is a fluid that can be completely characterized by its rest frame mass density ρ and isotropic pressure p.

Real fluids are "sticky" and contain (and conduct) heat. Perfect fluids are idealized models in which these possibilities are neglected. Specifically, perfect fluids have no shear stresses, viscosity, or heat conduction.

In tensor notation, the stress–energy tensor of a perfect fluid can be written in the form

T^{{\mu \nu }}=\left(\rho +{\frac {p}{c^{2}}}\right)\,U^{\mu }U^{\nu }+p\,\eta ^{{\mu \nu }}\,

where U is the velocity vector field of the fluid and where \eta _{{\mu \nu }} is the metric tensor of Minkowski spacetime.

Perfect fluids admit a Lagrangian formulation, which allows the techniques used in field theory, in particular, quantization, to be applied to fluids. This formulation can be generalized, but unfortunately, heat conduction and anisotropic stresses cannot be treated in these generalized formulations.

Perfect fluids are often used in general relativity to model idealized distributions of matter, such as in the interior of a star.

See also

References

External links

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.