Partial linear space

From Wikipedia, the free encyclopedia

A partial linear space (also semilinear or near-linear space) is a basic incidence structure in the field of incidence geometry, that carries slightly less structure than a linear space.

Definition

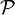Let $S=({{\mathcal P}},{{\mathcal B}},{\textbf {I}})$ an incidence structure, for which the elements of ${{\mathcal P}}$ are called points and the elements of 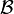${{\mathcal B}}$ are called lines. S is a partial linear space, if the following axioms hold:

any line is at least incident with two points
any pair of distinct points is incident with at most one line

References

Lynn Margaret Batten: Combinatorics of Finite Geometries. Cambridge University Press 1986, ISBN 0-521-31857-2, p. 1-22

External links

partial linear space at a website of the university of Kiel
partial linear space at PlanetMath

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.