Omnitruncated simplectic honeycomb

From Wikipedia, the free encyclopedia

In geometry an omnitruncated simplectic honeycomb or omnitruncated n-simplex honeycomb is an n-dimensional uniform tessellation, based on the symmetry of the {{\tilde {A}}}_{n} affine Coxeter group. Each is composed of omnitruncated simplex facets. The vertex figure for each is an irregular n-simplex.

The facets of an omnitruncated simplectic honeycomb are called permutahedra and can be positioned in n+1 space with integral coordinates, permutations of the whole numbers (0,1,..,n).

n {{\tilde {A}}}_{{1+}} Image Tessellation Facets Vertex figure Facets per vertex figure Vertices per vertex figure
1 {{\tilde {A}}}_{1} Apeirogon
 Line segment Line segment 1 2
2 {{\tilde {A}}}_{2} Hexagonal tiling
hexagon		 Equilateral triangle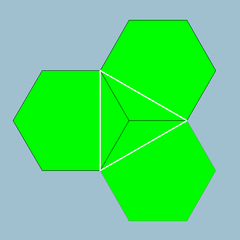
 3 hexagons 3
3 {{\tilde {A}}}_{3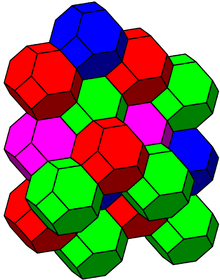} Bitruncated cubic honeycomb
Truncated octahedron		 irr. tetrahedron
 4 truncated octahedron 4
4 {{\tilde {A}}}_{4} Omnitruncated 4-simplex honeycomb
Omnitruncated 4-simplex		 irr. 5-cell
 5 omnitruncated 4-simplex 5
5 {{\tilde {A}}}_{5} Omnitruncated 5-simplex honeycomb
Omnitruncated 5-simplex		 irr. 5-simplex
 6 omnitruncated 5-simplex 6
6 {{\tilde {A}}}_{6} Omnitruncated 6-simplex honeycomb
Omnitruncated 6-simplex		 irr. 6-simplex
 7 omnitruncated 6-simplex 7
7 {{\tilde {A}}}_{7} Omnitruncated 7-simplex honeycomb
Omnitruncated 7-simplex		 irr. 7-simplex
 8 omnitruncated 7-simplex 8
8 {{\tilde {A}}}_{8} Omnitruncated 8-simplex honeycomb
Omnitruncated 8-simplex		 irr. 8-simplex
 9 omnitruncated 8-simplex 9

Projection by folding


The (2n-1)-simplex honeycombs can be projected into the n-dimensional omnitruncated hypercubic honeycomb by a geometric folding operation that maps two pairs of mirrors into each other, sharing the same vertex arrangement:

{{\tilde {A}}}_{3} {{\tilde {A}}}_{5} {{\tilde {A}}}_{7} {{\tilde {A}}}_{9} ...
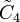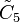{{\tilde {C}}}_{2} {{\tilde {C}}}_{3} {{\tilde {C}}}_{4} {{\tilde {C}}}_{5} ...

See also

References

  • George Olshevsky, Uniform Panoploid Tetracombs, Manuscript (2006) (Complete list of 11 convex uniform tilings, 28 convex uniform honeycombs, and 143 convex uniform tetracombs)
  • Branko Grünbaum, Uniform tilings of 3-space. Geombinatorics 4(1994), 49 - 56.
  • Norman Johnson Uniform Polytopes, Manuscript (1991)
  • Coxeter, H.S.M. Regular Polytopes, (3rd edition, 1973), Dover edition, ISBN 0-486-61480-8
  • Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6
    • (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I, [Math. Zeit. 46 (1940) 380-407, MR 2,10] (1.9 Uniform space-fillings)
    • (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III, [Math. Zeit. 200 (1988) 3-45]
Fundamental convex regular and uniform honeycombs in dimensions 2–11
Family {{\tilde {A}}}_{{n-1}} {{\tilde {C}}}_{{n-1}} {{\tilde {B}}}_{{n-1}} {{\tilde {D}}}_{{n-1}} {{\tilde {G}}}_{2} / {{\tilde {F}}}_{4} / {{\tilde {E}}}_{{n-1}}
Uniform tiling {3[3]} δ3 hδ3 qδ3 Hexagonal
Uniform convex honeycomb {3[4]} δ4 hδ4 qδ4
Uniform 5-honeycomb {3[5]} δ5 hδ5 qδ5 24-cell honeycomb
Uniform 6-honeycomb {3[6]} δ6 hδ6 qδ6
Uniform 7-honeycomb {3[7]} δ7 hδ7 qδ7 222
Uniform 8-honeycomb {3[8]} δ8 hδ8 qδ8 133 • 331
Uniform 9-honeycomb {3[9]} δ9 hδ9 qδ9 152 • 251 • 521
Uniform n-honeycomb {3[n]} δn hδn qδn 1k2 • 2k1 • k21
This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.