Omnitruncated 5-cell honeycomb

From Wikipedia, the free encyclopedia

Omnitruncated 4-simplex honeycomb
(No image)
Type	Uniform 4-honeycomb
Family	Omnitruncated simplectic honeycomb
Schläfli symbol	t_0,1,2,3,4{3^[5]}
Coxeter diagram
4-face types	t_0,1,2,3{3,3,3}
Cell types	t_0,1,2{3,3} {6}x{}
Face types	{4} {6}
Vertex figure	Irr. 5-cell
Symmetry	${{\tilde {A}}}_{4}$ ×10, [5[3^[5]]]
Properties	vertex-transitive, cell-transitive

In four-dimensional Euclidean geometry, the omnitruncated 4-simplex honeycomb or omnitruncated 5-cell honeycomb is a space-filling tessellation honeycomb. It is composed entirely of omnitruncated 5-cell (omnitruncated 4-simplex) facets.

Coxeter calls this Hinton's honeycomb after C. H. Hinton, who described it in his book The Fourth Dimension in 1906.^[1]

The facets of all omnitruncated simplectic honeycombs are called permutahedra and can be positioned in n+1 space with integral coordinates, permutations of the whole numbers (0,1,..,n).

Alernate names

Omnitruncated cyclopentachoric tetracomb
Great-prismatodecachoric tetracomb

A₄^* lattice

The A*
4 lattice is the union of five A₄ lattices, and is the dual to the omnitruncated 5-simplex honeycomb, and therefore the Voronoi cell of this lattice is an omnitruncated 5-cell

= dual of

Related polytopes and honeycombs

This honeycomb is one of seven unique uniform honeycombs^[2] constructed by the ${{\tilde {A}}}_{4}$ Coxeter group. The symmetry can be multiplied by the symmetry of rings in the Coxeter–Dynkin diagrams:

Pentagon symmetry	Extended symmetry	Extended order	Honeycomb diagrams
a1	[3^[5]]	×1	(None)
i2	[[3^[5]]]	×2	₁, ₂, ₃, ₄, ₅, ₆
r10	[5[3^[5]]]	×10	₇

Notes

↑ The Beauty of Geometry: Twelve Essays. Dover Publications. 1999. ISBN 0-486-40919-8. LCCN 99035678. (The classification of Zonohededra, page 73)
↑ , A000029 8-1 cases, skipping one with zero marks

References

Norman Johnson Uniform Polytopes, Manuscript (1991)
F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, ed. (1995). Kaleidoscopes: Selected Writings of H.S.M. Coxeter. Wiley-Interscience Publication. ISBN 978-0-471-01003-6.
- (Paper 22) Coxeter, H. S. M. (1940). "Regular and semi-regular polytopes. I". Mathematische Zeitschrift 46: 380. doi:10.1007/BF01181449. (1.9 Uniform space-fillings)
- (Paper 24) Coxeter, H. S. M. (1988). "Regular and semi-regular polytopes. III". Mathematische Zeitschrift 200: 3. doi:10.1007/BF01161745.
George Olshevsky, Uniform Panoploid Tetracombs, Manuscript (2006) (Complete list of 11 convex uniform tilings, 28 convex uniform honeycombs, and 143 convex uniform tetracombs) Model 140
Richard Klitzing, 4D, Euclidean tesselations, x3x3x3x3x3*a - otcypit - 140

v t e Fundamental convex regular and uniform honeycombs in dimensions 2–11
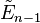Family	${{\tilde {A}}}_{{n-1}}$	${{\tilde {C}}}_{{n-1}}$	${{\tilde {B}}}_{{n-1}}$	${{\tilde {D}}}_{{n-1}}$	${{\tilde {G}}}_{2}$ / ${{\tilde {F}}}_{4}$ / ${{\tilde {E}}}_{{n-1}}$
Uniform tiling	{3^[3]}	δ₃	hδ₃	qδ₃	Hexagonal
Uniform convex honeycomb	{3^[4]}	δ₄	hδ₄	qδ₄
Uniform 5-honeycomb	{3^[5]}	δ₅	hδ₅	qδ₅	24-cell honeycomb
Uniform 6-honeycomb	{3^[6]}	δ₆	hδ₆	qδ₆
Uniform 7-honeycomb	{3^[7]}	δ₇	hδ₇	qδ₇	2₂₂
Uniform 8-honeycomb	{3^[8]}	δ₈	hδ₈	qδ₈	1₃₃ • 3₃₁
Uniform 9-honeycomb	{3^[9]}	δ₉	hδ₉	qδ₉	1₅₂ • 2₅₁ • 5₂₁
Uniform n-honeycomb	{3^[n]}	δ_n	hδ_n	qδ_n	1_k2 • 2_k1 • k₂₁

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.