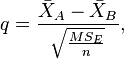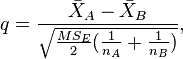Newman–Keuls method
The Newman–Keuls or Student–Newman–Keuls (SNK) method is a stepwise multiple comparisons procedure used to identify sample means that are significantly different from each other.[1] It was named after Student (1927),[2] D. Newman,[3] and M. Keuls.[4] This procedure is often used as a post-hoc test whenever a significant difference between three or more sample means has been revealed by an analysis of variance (ANOVA).[1] The Newman–Keuls method is similar to Tukey's range test as both procedures use Studentized range statistics.[5][6] Compared to Tukey's range test, the Newman–Keuls method is more powerful but less conservative.[6]
Procedure
The Newman–Keuls method employs a stepwise approach when comparing sample means.[7] Prior to any mean comparison, all sample means are rank-ordered in ascending or descending order, thereby producing an ordered range of sample means.[1][7] A comparison is then made between the largest and smallest sample means within the largest range.[7] Assuming that the largest range is four means, a significant difference between the largest and smallest means as revealed by the Newman–Keuls method would result in a rejection of the null hypothesis. The next largest comparison of two sample means would then be made within a smaller range of three means. Unless there is no significant differences between two sample means within any given range, this stepwise comparison of sample means will continue until a final comparison is made with the smallest range of just two means. If there is no significant difference between the two sample means, then all the null hypotheses within that range would be retained and no further comparisons within smaller ranges are necessary.
 |
 |
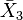 |
 | |
|---|---|---|---|---|
| Mean values | 2 | 4 | 6 | 8 |
| 2 | 2 | 4 | 6 | |
| 4 | 2 | 4 | ||
| 6 | 2 | |||
To determine if there is a significant difference between two means with equal sample sizes, the Newman–Keuls method uses a formula that is identical to the one used in Tukey's range test, which calculates the q value by taking the difference between two sample means and dividing it by the standard error:
where  represents the Studentized range value,
represents the Studentized range value,  and
and  are the largest and smallest sample means within a range,
are the largest and smallest sample means within a range, 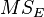 is the error variance taken from the ANOVA table, and
is the error variance taken from the ANOVA table, and  is the sample size (number of observations within a sample). The computed q value is then compared to a q critical value taken from a q distribution table. If the computed q value is equal to or greater than the q critical value, then the null hypothesis (H0: μA = μB) can be rejected.[8]
is the sample size (number of observations within a sample). The computed q value is then compared to a q critical value taken from a q distribution table. If the computed q value is equal to or greater than the q critical value, then the null hypothesis (H0: μA = μB) can be rejected.[8]
If comparisons are made with means of unequal sample sizes, then the Newman-Keuls formula would be adjusted as follows:
where 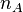 and
and 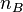 represent the sample sizes of the two sample means.
represent the sample sizes of the two sample means.
See also
- Multiple comparisons
- Post-hoc analysis
- Tukey's range test
References
- ↑ 1.0 1.1 1.2 Muth, James E. De (2006). Basic Statistics and Pharmaceutical Statistical Applications (2nd ed.). Boca Raton, FL: Chapman and Hall/CRC. pp. 229–259. ISBN 0-849-33799-2.
- ↑ Student (1927). "Errors of routine analysis". Biometrika 19 (1/2): 151–164.
- ↑ Newman D (1939). "The distribution of range in samples from a normal population, expressed in terms of an independent estimate of standard deviation". Biometrika 31 (1): 20–30. doi:10.1093/biomet/31.1-2.20.
- ↑ Keuls M (1952). "The use of the "studentized range" in connection with an analysis of variance". Euphytica 1: 112–122.
- ↑ Broota, K.D. (1989). Experimental Design in Behavioural Research (1st ed.). New Delhi, India: New Age International (P) Ltd. pp. 81–96. ISBN 8-122-40215-1.
- ↑ 6.0 6.1 Sheskin, David J. (1989). Handbook of Parametric and Nonparametric Statistical Procedures (3rd ed.). Boca Raton, FL: CRC Press. pp. 665–756. ISBN 1-584-88440-1.
- ↑ 7.0 7.1 7.2 Toothaker, Larry E. (1993). Multiple Comparison Procedures (Quantitative Applications in the Social Sciences) (2nd ed.). Newburry Park, CA: Chapman and Hall/CRC. pp. 27–45. ISBN 0-803-94177-3.
- ↑ Zar, Jerrold H. (1999). Biostatistical Analysis (4th ed.). Newburry Park, CA: Prentice Hall. pp. 208–230. ISBN 0-130-81542-X.