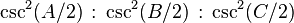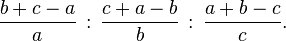Nagel point

From Wikipedia, the free encyclopedia
The Nagel point (blue, N) of a triangle (black). The red triangle is the extouch triangle, and the orange circles are the excircles

In geometry, the Nagel point is a point associated with any triangle. Given a triangle ABC, let TA, TB, and TC be the extouch points in which the A-excircle meets line BC, the B-excircle meets line CA, and C-excircle meets line AB, respectively. The lines ATA, BTB, CTC concur in the Nagel point N of triangle ABC. The Nagel point is named after Christian Heinrich von Nagel, a nineteenth-century German mathematician, who wrote about it in 1836.

Another construction of the point TA is to start at A and trace around triangle ABC half its perimeter, and similarly for TB and TC. Because of this construction, the Nagel point is sometimes also called the bisected perimeter point, and the segments ATA, BTB, CTC are called the triangle's splitters.

Relation to other triangle centers

The Nagel point is the isotomic conjugate of the Gergonne point. The Nagel point, the centroid, and the incenter are collinear on a line called the Nagel line. The incenter is the Nagel point of the medial triangle (Anonymous 1896); equivalently, the Nagel point is the incenter of the anticomplementary triangle.

Trilinear coordinates

Trilinear coordinates of the Nagel point were given by Gallatly (1913) as

\csc ^{2}(A/2)\,:\,\csc ^{2}(B/2)\,:\,\csc ^{2}(C/2)

or, equivalently, in terms of the side lengths a = |BC|, b = |CA|, and c = |AB|,

{\frac {b+c-a}{a}}\,:\,{\frac {c+a-b}{b}}\,:\,{\frac {a+b-c}{c}}.

See also

References

  • Anonymous; Hoover, William; Anthony, O. W (1896). Problem 73. "Geometry: 69-72". American Mathematical Monthly 3 (12): 329. doi:10.2307/2970994. JSTOR 2970994. 
  • Baptist, Peter (1987). "Historische Anmerkungen zu Gergonne- und Nagel-Punkt". Sudhoffs Archiv für Geschichte der Medizin und der Naturwissenschaften 71 (2): 230–233. MR 0936136. 
  • Gallatly, William (1913). The Modern Geometry of the Triangle (2nd ed.). London: Hodgson. pp. page 20. 

External links

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.