Moulton plane
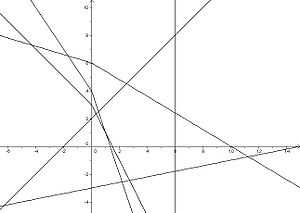
In incidence geometry, the Moulton plane is an example of an affine plane in which Desargues' theorem does not hold. It is named after the American astronomer Forest Ray Moulton. The points of the Moulton plane are simply the points in the real plane R2 and the lines are the regular lines as well with the exception, that for lines with a negative slope the slope doubles when they pass the y-axis.
Formal definition
The Moulton plane is an incidence structure  , where
, where  denotes the set of points,
denotes the set of points,  the set of lines and
the set of lines and  the incidence relation "lies on":
the incidence relation "lies on":
 is just a formal symbol for an element
is just a formal symbol for an element  . It is used to describe vertical lines, which you may think of as lines with an infinitely large slope.
. It is used to describe vertical lines, which you may think of as lines with an infinitely large slope.
The incidence relation is defined as follows:
For p = (x, y) ∈ P and g = (m, b) ∈ G we have
Application
The Moulton plane is an affine plane in which Desargues' theorem does not hold. The associated projective plane is consequently non-desarguesian as well. This means that there are projective planes not isomorphic to  for any (skew) field F. Here
for any (skew) field F. Here  is the projective plane
is the projective plane 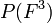 determined by a 3-dimensional vector space.
determined by a 3-dimensional vector space.
References
- Beutelspacher, A Rosenbaum, U. : Projektive Geometrie: Vieweg (1992), s. 70-71 (German)
- Moulton, Forest Ray (1902), "A Simple Non-Desarguesian Plane Geometry", Transactions of the American Mathematical Society (Providence, R.I.: American Mathematical Society) 3 (2): 192–195, ISSN 0002-9947, JSTOR 1986419


