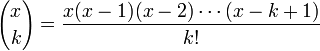Mahler's theorem

From Wikipedia, the free encyclopedia

Not to be confused with Mahler's compactness theorem.

In mathematics, Mahler's theorem, introduced by Kurt Mahler (1958), expresses continuous p-adic functions in terms of polynomials.

In any field, one has the following result. Let

$(\Delta f)(x)=f(x+1)-f(x)\,$

be the forward difference operator. Then for polynomial functions f we have the Newton series:

$f(x)=\sum _{{k=0}}^{\infty }(\Delta ^{k}f)(0){x \choose k},$

where

${x \choose k}={\frac {x(x-1)(x-2)\cdots (x-k+1)}{k!}}$

is the kth binomial coefficient polynomial.

Over the field of real numbers, the assumption that the function f is a polynomial can be weakened, but it cannot be weakened all the way down to mere continuity.

Mahler's theorem states that if f is a continuous p-adic-valued function on the p-adic integers then the same identity holds.

The relationship between the operator Δ and this polynomial sequence is much like that between differentiation and the sequence whose kth term is x^k.

It is remarkable that as weak an assumption as continuity is enough; by contrast, Newton series on the complex number field are far more tightly constrained, and require Carlson's theorem to hold.

It is a fact of algebra that if f is a polynomial function with coefficients in any field of characteristic 0, the same identity holds where the sum has finitely many terms.

References

Mahler, K. (1958), "An interpolation series for continuous functions of a p-adic variable", Journal für die reine und angewandte Mathematik 199: 23–34, ISSN 0075-4102, MR 0095821

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.