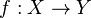Lusin's theorem

From Wikipedia, the free encyclopedia

This article is about the theorem of real analysis. For the separation thereom in descriptive set theory, see Lusin's separation theorem.

In the mathematical field of real analysis, Lusin's theorem (or Luzin's theorem, named for Nikolai Luzin) states that every measurable function is a continuous function on nearly all its domain. In the informal formulation of J. E. Littlewood, "every measurable function is nearly continuous".

Classical statement

For an interval [a, b], let

$f:[a,b]\rightarrow {\mathbb {C}}$

be a measurable function. Then, for every ε > 0, there exists a compact E ⊂ [a, b] such that f restricted to E is continuous and

$\mu (E)>b-a-\varepsilon .\,$

Note that E inherits the subspace topology from [a, b]; continuity of f restricted to E is defined using this topology.

General form

Let 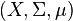$(X,\Sigma ,\mu )$ be a Radon measure space and Y be a second-countable topological space, let

$f:X\rightarrow Y$

be a measurable function. Given ε > 0, for every 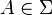$A\in \Sigma$ of finite measure there is a closed set E with µ(A \ E) < ε such that f restricted to E is continuous. If A is locally compact, we can choose E to be compact and even find a continuous function $f_{\varepsilon }:X\rightarrow Y$ with compact support that coincides with f on E.

Informally, measurable functions into spaces with countable base can be approximated by continuous functions on arbitrarily large portion of their domain.

A proof of Lusin's theorem

Since f is measurable, there exists a sequence of step functions, f_n converging to f pointwise almost everywhere. Each f_n is bounded on a set of finite measure, hence integrable. By Egorov's theorem, may take a closed set E, such that the measure of A \ E is arbitrarily small, and such that f_n converges to f uniformly. Thus f is in L¹(A). Since continuous functions are dense in L¹, we may approximate f with a continuous function defined on A.

References

N. Lusin. Sur les propriétés des fonctions mesurables, Comptes Rendus Acad. Sci. Paris 154 (1912), 1688-1690.

G. Folland. Real Analysis: Modern Techniques and Their Applications, 2nd ed. Chapter 2

W. Zygmunt. Scorza-Dragoni property (in Polish), UMCS, Lublin, 1990

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.