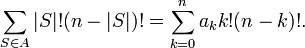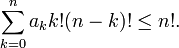Lubell–Yamamoto–Meshalkin inequality
In combinatorial mathematics, the Lubell–Yamamoto–Meshalkin inequality, more commonly known as the LYM inequality, is an inequality on the sizes of sets in a Sperner family, proved by Bollobás (1965), Lubell (1966), Meshalkin (1963), and Yamamoto (1954). It is named for the initials of three of its discoverers.
This inequality belongs to the field of combinatorics of sets, and has many applications in combinatorics. In particular, it can be used to prove Sperner's theorem. Its name is also used for similar inequalities.
Statement of the theorem
Let U be an n-element set, let A be a family of subsets of U such that no set in A is a subset of another set in A, and let ak denote the number of sets of size k in A. Then
Lubell's proof
Lubell (1966) proves the Lubell–Yamamoto–Meshalkin inequality by a double counting argument in which he counts the permutations of U in two different ways. First, by counting all permutations of U directly, one finds that there are n! of them. But secondly, one can generate a permutation of U by selecting a set S in A and concatenating a permutation of the elements of S with a permutation of the nonmembers. If |S| = k, it will be associated in this way with k!(n − k)! permutations. Each permutation can only be associated with a single set in A, for if two prefixes of a permutation both formed sets in A then one would be a subset of the other. Therefore, the number of permutations that can be generated by this procedure is
Since this number is at most the total number of all permutations,
Finally dividing the above inequality by n! leads to the result.
References
- Bollobás, B. (1965), "On generalized graphs", Acta Mathematica Academiae Scientiarum Hungaricae 16 (3–4): 447–452, doi:10.1007/BF01904851, MR 0183653.
- Lubell, D. (1966), "A short proof of Sperner's lemma", Journal of Combinatorial Theory 1 (2): 299, doi:10.1016/S0021-9800(66)80035-2, MR 0194348.
- Meshalkin, L. D. (1963), "Generalization of Sperner's theorem on the number of subsets of a finite set", Theory of Probability and its Applications 8 (2): 203–204, doi:10.1137/1108023, MR 0150049.
- Yamamoto, Koichi (1954), "Logarithmic order of free distributive lattice", Journal of the Mathematical Society of Japan 6: 343–353, MR 0067086.