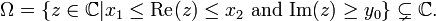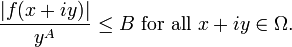Lindelöf's theorem

From Wikipedia, the free encyclopedia

In mathematics, Lindelöf's theorem is a result in complex analysis named after the Finnish mathematician Ernst Leonard Lindelöf. It states that a holomorphic function on a half-strip in the complex plane that is bounded on the boundary of the strip and does not grow "too fast" in the unbounded direction of the strip must remain bounded on the whole strip. The result is useful in the study of the Riemann zeta function, and is a special case of the Phragmén–Lindelöf principle. Also, see Hadamard three-lines theorem.

Statement of the theorem

Let Ω be a half-strip in the complex plane:

$\Omega =\{z\in {\mathbb {C}}|x_{1}\leq {\mathrm {Re}}(z)\leq x_{2}{\text{ and }}{\mathrm {Im}}(z)\geq y_{0}\}\subsetneq {\mathbb {C}}.\,$

Suppose that ƒ is holomorphic (i.e. analytic) on Ω and that there are constants M, A and B such that

$|f(z)|\leq M{\text{ for all }}z\in \partial \Omega \,$

and

${\frac {|f(x+iy)|}{y^{{A}}}}\leq B{\text{ for all }}x+iy\in \Omega .\,$

Then f is bounded by M on all of Ω:

$|f(z)|\leq M{\text{ for all }}z\in \Omega .\,$

Proof

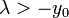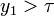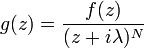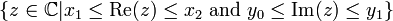Fix a point $\xi =\sigma +i\tau$ inside $\Omega$ . Choose $\lambda >-y_{0}$ , an integer $N>A$ and $y_{1}>\tau$ large enough such that ${\frac {By_{1}^{A}}{(y_{1}+\lambda )^{N}}}\leq {\frac {M}{(y_{0}+\lambda )^{N}}}$ . Applying maximum modulus principle to the function $g(z)={\frac {f(z)}{(z+i\lambda )^{N}}}$ and the rectangular area $\{z\in {\mathbb {C}}|x_{1}\leq {\mathrm {Re}}(z)\leq x_{2}{\text{ and }}y_{0}\leq {\mathrm {Im}}(z)\leq y_{1}\}$ we obtain 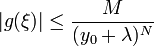$|g(\xi )|\leq {\frac {M}{(y_{0}+\lambda )^{N}}}$ , that is, $|f(\xi )|\leq M\left({\frac {|\xi +\lambda |}{y_{0}+\lambda }}\right)^{N}$ . 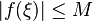Letting $\lambda \rightarrow +\infty$ yields $|f(\xi )|\leq M$ as required.

References

Edwards, H.M. (2001). Riemann's Zeta Function. New York, NY: Dover. ISBN 0-486-41740-9.

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.