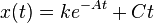Leaky integrator

From Wikipedia, the free encyclopedia

A graph of a leaky integrator; the input changes at T=5.

In mathematics, a leaky integrator equation is a specific differential equation, used to describe a component or system that takes the integral of an input, but gradually leaks a small amount of input over time. It appears commonly in hydraulics, electronics, and neuroscience where it can represent either a single neuron or a local population of neurons.^[1]

Equation

The equation is of the form

$dx/dt=-Ax+C\,$

where C is the input and A is the rate of the 'leak'.

General solution

Its general solution is

$x(t)=ke^{{-At}}+Ct\,$

where k is a constant.

References

↑ Eliasmith, Anderson, Chris, Charles (2003). Neural Engineering. Cambridge, Massachusetts: MIT Press. p. 81.

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.