Kleinian model
From Wikipedia, the free encyclopedia
Main article: Beltrami–Klein model
In mathematics, a Kleinian model is a model of a three-dimensional hyperbolic manifold N by the quotient space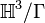 
where Γ is a discrete subgroup of PSL(2,C). Here, the subgroup Γ, a Kleinian group, is defined so that it is isomorphic to the fundamental group 
of the surface N. Many authors use the terms Kleinian group and Kleinian model interchangeably, letting one stand for the other.
Many properties of Kleinian models are in direct analogy to those of Fuchsian models; however, overall, the theory is less well developed. A number of unsolved conjectures on Kleinian models are the analogs to theorems on Fuchsian models.
See also
References
This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.