Interval vector

Example of Z-relation on two pitch sets analyzable as or derivable from Z17^[2]  Play (help·info), with intervals between pitch classes labeled for ease of comparison between the two sets and their common interval vector, 212320.

Interval vector: C major chord {0,4,7}: 001110.

In musical set theory, an interval vector (also called an interval-class vector or ic vector) is an array that expresses the intervallic content of a pitch-class set. Often referred to as a PIC vector (or pitch-class interval vector), Schuijer suggests that APIC vector (or absolute pitch-class interval vector) is more accurate.

One can think of the ICV as the common calculus derivative of the source material as a discrete function; ICV is at root a vector: a non-scalar value in simple math, and thus subject to the universe of mathematics. The interval vector is a species of integer vector calculus differential, a vector differential of the source material taken as a binary vector.^{[citation needed]} It can also be calculated via a sort of Discrete Fourier transform using the Integer function in place of the Exponential function. Exactly as Fourier transform maps a waveform between time domain and harmonic-content domain, the ICV maps between an applied musical domain and an harmonic-reductionist domain.^{[citation needed]}

In 12 equal temperament the ICV has six digits, with each digit standing for the number of times an interval class appears in the set. (Interval classes, not regular intervals, must be used, in order that the interval vector remains the same, regardless of the set's permutation or vertical arrangement.) The interval classes represented by each digit ascend from left to right. That is:

1) minor seconds/major sevenths (1 or 11 semitones)

2) major seconds/minor sevenths (2 or 10 semitones)

3) minor thirds/major sixths (3 or 9 semitones)

4) major thirds/minor sixths (4 or 8 semitones)

5) perfect fourths/perfect fifths (5 or 7 semitones)

6) tritones (6 semitones) (The tritone is inversionally related to itself.)

Interval class 0 (representing unisons and octaves) is omitted.

The concept was named intervalic content by Howard Hanson in his The Harmonic Materials of Modern Music, where he introduced the monomial notation p^em^dn^c.s^bd^at^{f [note 1]} for what would now be written <a b c d e f>. The modern notation, which has considerable advantages and is extendable to any equal division of the octave was introduced by Allen Forte.

A scale whose interval vector contains six different numbers is said to have the deep scale property. Major, natural minor and modal scales have this property.

For a practical example, the interval vector for a C major triad in the root position, {C E G} ( Play (help·info)), is <001110>. This means that the set has one major third or minor sixth (i.e. from C to E, or E to C), one minor third or major sixth (i.e. from E to G, or G to E), and one perfect fifth or perfect fourth (i.e. from C to G, or G to C). As the interval vector will not change with transposition or inversion, it belongs to the entire set class, and <001110> is the vector of all major (and minor) triads. It should, however, be noted that some interval vectors correspond to more than one sets that cannot be transposed or inverted to produce the other. (These are called Z-related sets, explained below).

For a set of x elements, the sum of all the numbers in the set's interval vector equals (x*(x-1))/2.

While primarily an analytic tool, interval vectors can also be useful for composers, as they quickly show the sound qualities that are created by different collections of pitch classes. That is, sets with high concentrations of conventionally dissonant intervals (i.e. seconds and sevenths) will generally be heard as more dissonant, while sets with higher numbers of conventionally consonant intervals (i.e. thirds and sixths) will be heard as more consonant. (While the actual perception of consonance and dissonance involves many contextual factors, such as register, an interval vector, nevertheless, can be a helpful tool.)

An expanded form of the interval vector is also used in transformation theory, as set out in David Lewin's Generalized Musical Intervals and Transformations.

Z-relation

Successive Z-related hexachords from act 3 of Wozzeck^[1]  Play (help·info).

In musical set theory, a Z-relation, also called isomeric relation, is a relation between two pitch-class sets in which the two sets have the same intervallic content (i.e. they have the same interval vector), but they are of different T_n-type and T_n/T_nI-type. That is to say, one set cannot be derived from the other through transposition or inversion.^[2]

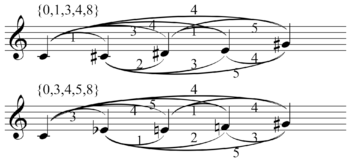
For example, the two sets {0,1,4,6} and {0,1,3,7} have the same interval vector (<1,1,1,1,1,1>) but they are not transpositionally or inversionally related.

In the case of hexachords each may be referred to as a Z-hexachord. Any hexachord not of the "Z" type is its own complement while the complement of a Z-hexachord is its Z-correspondent, for example 6-Z3 and 6-Z36.^[1] See: 6-Z44, 6-Z17, 6-Z11, and Forte number.

The term, for "zygotic" (yoked or the fusion of two reproductive cells),^[3] originated with Allen Forte in 1964, but the notion seems to have first been considered by Howard Hanson. Hanson termed this the isomeric relationship, defining two such sets to be isomeric.^[4] According to Michael Schuijer (2008), "the discovery of the relation," was, "reported," by David Lewin in 1960.^[3][5]

Though it is commonly observed that Z-related sets always occur in pairs, David Lewin noted that this is a result of twelve-tone equal temperament (12-ET). In 16-ET, Z-related sets are found as triplets. Lewin's student Jonathan Wild continued this work for other tuning systems, finding Z-related tuplets with up to 16 members in higher ET systems.

Straus argues, "[sets] in the Z-relation will sound similar because they have the same interval content."^[6] Some argue that the "relation" is often so remote as to be imperceptible, but certain composers have exploited the Z-relation in their work. For instance, the play between {0,1,4,6} and {0,1,3,7} is clear in Elliot Carter's second string quartet.

Multiplication

Some Z-related chords are connected by M or IM (multiplication by 5 or multiplication by 7), due to identical entries for 1 and 5 on the interval vector.^[3]

See also

• Interval cycle
• Pitch interval

Further reading

• Rahn, John (1980). Basic Atonal Theory. ISBN 0-02-873160-3.

Notes

1. ↑ In order to quantify the consonant/dissonant content of a set, Hanson ordered the intervals according to their dissonance degree, with p=perfect fifth, m=major third, n=minor third, s=major second, d=(more dissonant) minor second, t=tritone

Sources

1. ↑ 1.0 1.1 Forte, Allen (1977). The Structure of Atonal Music, p.79. Yale University Press. ISBN 0-300-02120-8.
2. ↑ 2.0 2.1 Schuijer, Michael (2008). Analyzing Atonal Music: Pitch-Class Set Theory and Its Contexts, p.99. ISBN 978-1-58046-270-9.
3. ↑ 3.0 3.1 3.2 Schuijer (2008), p.98 and 98n18. The meaning of "Z" was finally revealed on Nov. 17, 2004.
4. ↑ Hanson, Howard (1960). Harmonic Materials of Modern Music, . Appleton-Century-Crofts. ISBN 0-89197-207-2.
5. ↑ Lewin, David. "The Intervallic Content of a Collection of Notes, Intervallic Relations between a Collection of Notes and its Complement: an Application to Schoenberg’s Hexachordal Pieces." Journal of Music Theory 4/1 (1960): 98–101.
6. ↑ Straus (1990). Introduction to Post-Tonal Theory, 67. ISBN 0-13-189890-6. Cited in Schuijer (2008), p.125.

External links

• Set classes and interval-class content
• Introduction to Post-Functional Music Analysis: Post-Functional Theory Terminology by Robert T. Kelley
• Twentieth Century Pitch Theory: Some Useful Terms and Techniques