Interval order

From Wikipedia, the free encyclopedia

In mathematics, especially order theory, the interval order for a collection of intervals on the real line is the partial order corresponding to their left-to-right precedence relation—one interval, I₁, being considered less than another, I₂, if I₁ is completely to the left of I₂. 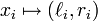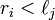More formally, a poset $P=(X,\leq )$ is an interval order if and only if there exists a bijection from $X$ to a set of real intervals, so $x_{i}\mapsto (\ell _{i},r_{i})$ , such that for any $x_{i},x_{j}\in X$ we have $x_{i}<x_{j}$ in $P$ exactly when $r_{i}<\ell _{j}$ .

An interval order defined by unit intervals is a semiorder.

The complement of the comparability graph of an interval order ( $X$ , ≤) is the interval graph $(X,\cap )$ .

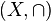Interval orders should not be confused with the interval-containment orders, which are the containment orders on intervals on the real line (equivalently, the orders of dimension ≤ 2).

Interval dimension

The interval dimension of a partial order can be defined as the minimal number of interval order extensions realizing this order, in a similar way to the definition of the order dimension which uses linear extensions. The interval dimension of an order is always less than its order dimension,^[1] but interval orders with high dimensions are known to exist. While the problem of determining the order dimension of general partial orders is known to be NP-complete, the complexity of determining the order dimension of an interval order is unknown.^[2]

References

Fishburn, Peter (1985). Interval Orders and Interval Graphs: A Study of Partially Ordered Sets. John Wiley.
Gunther Schmidt, 2010. Relational Mathematics. Cambridge University Press, ISBN 978-0-521-76268-7.

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.