Infinitesimal calculus

From Wikipedia, the free encyclopedia

Isaac Newton (left) and Gottfried Wilhelm Leibniz (right), developers of infinitesimal calculus

Infinitesimal calculus is the part of mathematics concerned with finding tangent lines to curves, areas under curves, minima and maxima, and other geometric and analytic problems.

History

Founders

Infinitesimal calculus was independently developed by Gottfried Leibniz and Isaac Newton starting in the 1660s. John Wallis refined earlier techniques of indivisibles of Cavalieri and others by exploiting an infinitesimal quantity he denoted ${\tfrac {1}{\infty }}$ in area calculations, preparing the ground for integral calculus.^[1] They drew on the work of such mathematicians as Pierre de Fermat (see adequality), Isaac Barrow and René Descartes. Infinitesimal calculus consists of differential calculus and integral calculus, respectively used for the techniques of differentiation and integration.

Newton sought to remove the use of infinitesimals from his fluxional calculus, preferring to talk of velocities as in "For by the ultimate velocity is meant ... the ultimate ratio of evanescent quantities". Leibniz embraced the concept fully calling differentials "...an evanescent quantity which yet retains the character of that which is disappearing", and developed versatile heuristic principles such as the Law of Continuity and the Transcendental law of homogeneity to manipulate them. Leibniz's notation for them is the current symbolism in calculus.

Further development

In early calculus the use of infinitesimal quantities was criticized by a number of authors, most notably Michel Rolle and Bishop Berkeley. Berkeley mocked infinitesimals in his book The Analyst in 1734. Kirsti Andersen (2011) showed that Berkeley's doctrine of the compensation of errors in The Analyst contains a logical circularity. Namely, Berkeley relies upon Apollonius's determination of the tangent of the parabola in Berkeley's determination of the derivative of the quadratic function. A recent study argues that the force of Berkeley's criticisms has been overestimated; that Leibniz's defense of infinitesimals is more firmly grounded than Berkeley's criticism thereof; and that Leibniz's system for differential calculus was free of logical contradictions.^[2]

Several mathematicians, including Maclaurin and d'Alembert, advocated the use of limits. Augustin Louis Cauchy developed a versatile spectrum of foundational approaches, including a definition of continuity in terms of infinitesimals and a (somewhat imprecise) prototype of an ε, δ argument in working with differentiation. Karl Weierstrass formalized the concept of limit in the context of a (real) number system without infinitesimals. Following the work of Weierstrass, it eventually became common to base calculus on ε, δ arguments instead of infinitesimals.

This approach formalized by Weierstrass came to be known as the standard calculus. Informally, the expression "infinitesimal calculus" is commonly used to refer to Weierstrass' approach but has become something of a dead metaphor.^[3]

Modern infinitesimals

Main article: Hyperreal number

After many years of the infinitesimal approach to calculus having fallen into disuse other than as an introductory pedagogical tool, use of infinitesimal quantities was finally given a rigorous foundation by Abraham Robinson in the 1960s. Robinson's approach, called non-standard analysis, uses technical machinery from mathematical logic to create a theory of hyperreal numbers that interpret infinitesimals in a manner that allows a Leibniz-like development of the usual rules of calculus. An alternative approach, developed by Edward Nelson, finds infinitesimals on the ordinary real line itself, and involves a modification of the foundational setting by enriching ZFC through the introduction of a new unary predicate "standard".

Varieties

Differential calculus (left) and Integral calculus (right).

Differential calculus

Main article: Differential calculus

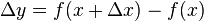Differential calculus is a subfield of calculus concerned with the study of the rates at which quantities change. Here the fundamental concept is that of a derivative. Given a function $y=f(x)$ , the derivative ${\frac {dy}{dx}}$ is defined as follows. Choose an infinitesimal increment $\Delta x$ of the dependent variable x, and compute the corresponding change $\Delta y=f(x+\Delta x)-f(x)$ of the dependent variable y. 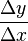The derivative ${\frac {dy}{dx}}$ is then the ratio ${\frac {\Delta y}{\Delta x}}$ rounded off to the nearest real number. In more detail, 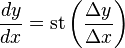${\frac {dy}{dx}}={\text{st}}\left({\frac {\Delta y}{\Delta x}}\right)$ where "st" denotes the standard part function (sometimes referred to as the shadow). 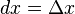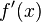Here one can set $dx=\Delta x$ and $dy=f'(x)dx$ where $f'(x)$ is Lagrange's notation for the derivative, so that ${\frac {dy}{dx}}$ is a true ratio.

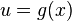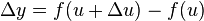If $u=g(x)$ is a dependent variable and $y=f(u)$ then an infinitesimal $\Delta x$ produces a dependent variable $\Delta u=g(x+\Delta x)-g(x)$ . If $\Delta u$ is nonzero, one can use it to calculate $\Delta y=f(u+\Delta u)-f(u)$ , producing the relation 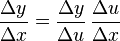${\frac {\Delta y}{\Delta x}}={\frac {\Delta y}{\Delta u}}\,{\frac {\Delta u}{\Delta x}}$ . Applying standard part to this relation, we obtain the chain rule ${\frac {dy}{dx}}={\frac {dy}{du}}\,{\frac {du}{dx}}$ for the function $f\circ g$ .

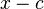The halo (or monad) $\mu (c)$ of a point $c$ consists of points $x$ infinitely close to $c$ (i.e., $x-c$ is infinitesimal). A function $f$ on ${\mathbb {R}}$ is continuous at a real point $c$ if and only if $f(\mu (c))\subset \mu (f(c))$ .

Integral calculus

Integral calculus is defined informally to be the area of the region in the xy-plane bounded by the graph of f, the x-axis, and the vertical lines x = a and x = b, such that areas above the axis add to the total, and the area below the x axis subtract from the total. The integral is defined as the standard part of an infinite Riemann sum associated with a partition of the domain of integration into infinitesimal subintervals.

Limit of the function at infinity.

Standard calculus

Main article: Calculus

Standard calculus is based on the approach that Weierstrass took, replacing infinitesimals by limits. Limits describe the value of a function at a certain input in terms of its values at nearby input. They capture small-scale behavior, just like infinitesimals, but use the real number system. In this treatment, calculus is a collection of techniques for manipulating certain limits. Infinitesimals get replaced by very small numbers, and the infinitely small behavior of the function is found by taking the limiting behavior for smaller and smaller numbers. Limits are the easiest way to provide rigorous foundations for calculus, and for this reason they are the standard approach.

Non-standard calculus

Main article: Non-standard calculus

Calculations with infinitesimals were widely replaced with the (ε, δ)-definition of limit starting in the 1870s. For almost one hundred years thereafter, mathematicians like Richard Courant viewed infinitesimals as being naive and vague or meaningless.^[4]

Contrary to such views, Abraham Robinson in 1960 developed precise, clear, and meaningful rules for working with infinitesimals, building upon work by Edwin Hewitt and Jerzy Łoś. According to Jerome Keisler, "Robinson solved a three hundred year old problem by giving a precise treatment of infinitesimals. Robinson's achievement will probably rank as one of the major mathematical advances of the twentieth century."^[5]

Smooth infinitesimal analysis

Main article: Smooth infinitesimal analysis

This is a mathematically rigorous reformulation of the calculus in terms of infinitesimals. Based on the ideas of F. W. Lawvere and employing the methods of category theory, it views all functions as being continuous and incapable of being expressed in terms of discrete entities. As a theory, it is a subset of synthetic differential geometry.

References

Notes

↑ Scott, J.F. 1981. "The Mathematical Work of John Wallis, D.D., F.R.S. (1616–1703)". Chelsea Publishing Co. New York, NY. p. 18.
↑ Katz, Mikhail; Sherry, David (2012), "Leibniz’s Infinitesimals: Their Fictionality, Their Modern Implementations, and Their Foes from Berkeley to Russell and Beyond", Erkenntnis, arXiv:1205.0174, doi:10.1007/s10670-012-9370-y
↑ Katz, Mikhail; Tall, David (2011), Tension between Intuitive Infinitesimals and Formal Mathematical Analysis, Bharath Sriraman, Editor. Crossroads in the History of Mathematics and Mathematics Education. The Montana Mathematics Enthusiast Monographs in Mathematics Education 12, Information Age Publishing, Inc., Charlotte, NC, arXiv:1110.5747
↑ Courant described infinitesimals on page 81 of Differential and Integral Calculus, Vol I, as "devoid of any clear meaning" and "naive befogging". Similarly on page 101, Courant described them as "incompatible with the clarity of ideas demanded in mathematics", "entirely meaningless", "fog which hung round the foundations", and a "hazy idea".
↑ Elementary Calculus: An Infinitesimal Approach

Other

Andersen, Kirsti (2011). "One of Berkeley's arguments on compensating errors in the calculus". Historia Mathematica 38 (2): 219–231. doi:10.1016/j.hm.2010.07.001.
Baron, Margaret E.: The origins of the infinitesimal calculus. Dover Publications, Inc., New York, 1987.
Baron, Margaret E.: The origins of the infinitesimal calculus. Pergamon Press, Oxford-Edinburgh-New York 1969. (A new edition of Baron's book appeared in 2004)
Hazewinkel, Michiel, ed. (2001), "Infinitesimal calculus", Encyclopedia of Mathematics, Springer, ISBN 978-1-55608-010-4
Lavendhomme, R.: Basic concepts of synthetic differential geometry, Kluwer, Dordrecht, 1996
O'Connor, Michael: An Introduction to Smooth Infinitesimal Analysis

v t e Infinitesimals

History	Adequality Infinitesimal calculus Leibniz's notation Integral symbol Criticism of non-standard analysis The Analyst The Method of Mechanical Theorems Cavalieri's principle

Related branches	Non-standard analysis Non-standard calculus Internal set theory Synthetic differential geometry Constructive non-standard analysis

Formalizations	Differentials Hyperreal numbers Dual numbers Surreal numbers

Individual concepts	Standard part function Transfer principle Hyperinteger Increment theorem Monad Internal set Levi-Civita field Hyperfinite set Law of Continuity Overspill Microcontinuity Transcendental law of homogeneity

Scientists	Gottfried Wilhelm Leibniz Abraham Robinson Pierre de Fermat Augustin-Louis Cauchy Leonhard Euler

Physics Engineering	Infinitesimal strain theory Infinitesimal oscillations of a pendulum

Textbooks	Analyse des Infiniment Petits Elementary Calculus Cours d'Analyse

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.