Hermitian wavelet

From Wikipedia, the free encyclopedia

Hermitian wavelets are a family of continuous wavelets, used in the continuous wavelet transform. The $n^{{\textrm {th}}}$ Hermitian wavelet is defined as the $n^{{\textrm {th}}}$ derivative of a Gaussian:

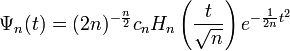
$\Psi _{{n}}(t)=(2n)^{{-{\frac {n}{2}}}}c_{{n}}H_{{n}}\left({\frac {t}{{\sqrt {n}}}}\right)e^{{-{\frac {1}{2n}}t^{{2}}}}$

where $H_{{n}}\left({x}\right)$ denotes the $n^{{\textrm {th}}}$ Hermite polynomial.

The normalisation coefficient 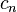$c_{{n}}$ is given by:

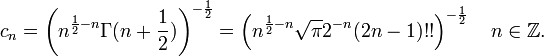
$c_{{n}}=\left(n^{{{\frac {1}{2}}-n}}\Gamma (n+{\frac {1}{2}})\right)^{{-{\frac {1}{2}}}}=\left(n^{{{\frac {1}{2}}-n}}{\sqrt {\pi }}2^{{-n}}(2n-1)!!\right)^{{-{\frac {1}{2}}}}\quad n\in {\mathbb {Z}}.$

The prefactor $C_{{\Psi }}$ in the resolution of the identity of the continuous wavelet transform for this wavelet is given by:

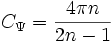
$C_{{\Psi }}={\frac {4\pi n}{2n-1}}$

i.e. Hermitian wavelets are admissible for all positive $n$ .

In computer vision and image processing, Gaussian derivative operators of different orders are frequently used as a basis for expressing various types of visual operations; see scale space and N-jet.

Examples of Hermitian wavelets: Starting from a Gaussian function with 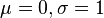$\mu =0,\sigma =1$ :

$f(t)=\pi ^{{-1/4}}e^{{(-t^{2}/2)}}$

the first 3 derivatives read

${\begin{aligned}f'(t)&=-\pi ^{{-1/4}}te^{{(-t^{2}/2)}}\\f''(t)&=\pi ^{{-1/4}}(t^{2}-1)e^{{(-t^{2}/2)}}\\f^{{(3)}}(t)&=\pi ^{{-1/4}}(3t-t^{3})e^{{(-t^{2}/2)}}\end{aligned}}$

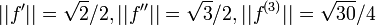
and their $L^{2}$ norms $||f'||={\sqrt {2}}/2,||f''||={\sqrt {3}}/2,||f^{{(3)}}||={\sqrt {30}}/4$

So the wavelets which are the negative normalized derivatives are:

${\begin{aligned}\Psi _{{1}}(t)&={\sqrt {2}}\pi ^{{-1/4}}te^{{(-t^{2}/2)}}\\\Psi _{{2}}(t)&={\frac {2}{3}}{\sqrt {3}}\pi ^{{-1/4}}(1-t^{2})e^{{(-t^{2}/2)}}\\\Psi _{{3}}(t)&={\frac {2}{15}}{\sqrt {30}}\pi ^{{-1/4}}(t^{3}-3t)e^{{(-t^{2}/2)}}\end{aligned}}$

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.