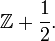Half-integer

From Wikipedia, the free encyclopedia

In mathematics, a half-integer is a number of the form

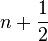$n+{1 \over 2}$ ,

where $n$ is an integer. For example,

4½, 7/2, −13/2, 8.5

are all half-integers. Note that a half of an integer is not always a half-integer: half of an even integer is an integer but not a half-integer. The half-integers are precisely those numbers that are half of an odd integer.

The set of all half-integers is often denoted

${\mathbb Z}+{1 \over 2}.$

Uses

Half-integers occur frequently enough in mathematical contexts that a special term for them is convenient. For example, the densest lattice packing of unit spheres in four dimensions places a sphere at every point whose coordinates are either all integers or all half-integers; this packing is closely related to the Hurwitz integers, which are quaternions whose real coefficients are either all integers or all half-integers.

Moreover, the Pauli exclusion principle results from definition of fermions as particles which have spins that are half-integers. The energy levels of the quantum harmonic oscillator occur at half-integers and thus its lowest energy is not zero.

Also, the factorial and gamma functions, while not defined for negative and non-positive integers respectively, are defined for all half-integers as rational multiples of the square root of pi.

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.