Friedlander–Iwaniec theorem

From Wikipedia, the free encyclopedia

In analytic number theory, an advanced branch of mathematics, the Friedlander–Iwaniec theorem[1] asserts that there are infinitely many prime numbers of the form 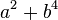a^{2}+b^{4}. The first few such primes are

2, 5, 17, 37, 41, 97, 101, 137, 181, 197, 241, 257, 277, 281, 337, 401, 457, 577, 617, 641, 661, 677, 757, 769, 821, 857, 881, 977, … (sequence A028916 in OEIS).

The theorem was proved in 1997 by John Friedlander and Henryk Iwaniec,[2] using sieve techniques first developed by Enrico Bombieri. Iwaniec was awarded the 2001 Ostrowski Prize in part for his contributions to this work.[3]

This result, however, does not imply that there are an infinite number of primes of form a^{2}+1, or

2, 5, 17, 37, 101, 197, 257, 401, 577, 677, 1297, 1601, 2917, 3137, 4357, 5477, 7057, 8101, 8837, 12101, 13457, 14401, 15377, … (sequence A002496 in OEIS).

as the latter is still an unsolved problem.

References

  1. ↑ van Golstein Brouwers, G.; Bamberg, D.; Cairns, J. (2004), "Totally Goldbach numbers and related conjectures", Australian Mathematical Society Gazette 31 (4): 251–255 [p. 254] .
  2. ↑ Friedlander, John; Iwaniec, Henryk (1997), "Using a parity-sensitive sieve to count prime values of a polynomial", PNAS 94 (4): 1054–1058, doi:10.1073/pnas.94.4.1054, PMC 19742, PMID 11038598 .
  3. ↑ "Iwaniec, Sarnak, and Taylor Receive Ostrowski Prize"

Further reading

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.