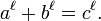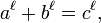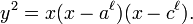Frey curve

From Wikipedia, the free encyclopedia

In mathematics, a Frey curve or Frey–Hellegouarch curve is the elliptic curve

$y^{2}=x(x-a^{\ell })(x+b^{\ell })\$

associated with a solution of Fermat's equation

$a^{\ell }+b^{\ell }=c^{\ell }.\$

History

Yves Hellegouarch (1975) came up with the idea of associating solutions (a,b,c) of Fermat's equation with a completely different mathematical object: an elliptic curve. If ℓ is an odd prime and a, b, and c are positive integers such that

$a^{\ell }+b^{\ell }=c^{\ell },\$

then a corresponding Frey curve is an algebraic curve given by the equation

$y^{2}=x(x-a^{\ell })(x+b^{\ell })\$

or, equivalently

$y^{2}=x(x-a^{\ell })(x-c^{\ell }).\$

This is a nonsingular algebraic curve of genus one defined over Q, and its projective completion is an elliptic curve over Q.

(Gerhard Frey 1982) called attention to the unusual properties of the same curve as Hellegouarch, which became called a Frey curve. This provided a bridge between Fermat and Taniyama by showing that a counterexample to Fermat's Last Theorem would create such a curve that would not be modular. The conjecture attracted considerable interest when Frey (1986) suggested that the Taniyama–Shimura–Weil conjecture implies Fermat's Last Theorem. However, his argument was not complete. In 1985, Jean-Pierre Serre proposed that a Frey curve could not be modular and provided a partial proof of this. This showed that a proof of the semistable case of the Taniyama-Shimura conjecture would imply Fermat's Last Theorem. Serre did not provide a complete proof and what was missing became known as the epsilon conjecture or ε-conjecture. In the summer of 1986, Ribet (1990) proved the epsilon conjecture, thereby proving that the Taniyama–Shimura–Weil conjecture implied Fermat's Last Theorem.

References

Frey, Gerhard (1986), "Links between stable elliptic curves and certain Diophantine equations", Annales Universitatis Saraviensis. Series Mathematicae 1 (1): iv+40, ISSN 0933-8268, MR 853387
Frey, Gerhard (1982), "Rationale Punkte auf Fermatkurven und getwisteten Modulkurven", J.reine u.angew.Math 331: 185–191
Hellegouarch, Yves (2000), "Rectificatif à l'article de H. Darmon intitulé : "La Conjecture de Shimura-Taniyama-Weil est enfin démontré"", Gazette des Mathématiciens 83, ISSN 0224-8999
Hellegouarch, Yves (1974), "Points d'ordre 2p^h sur les courbes elliptiques", Polska Akademia Nauk. Instytut Matematyczny. Acta Arithmetica 26 (3): 253–263, ISSN 0065-1036, MR 0379507
Hellegouarch, Yves (2002), Invitation to the mathematics of Fermat-Wiles, Boston, MA: Academic Press, ISBN 978-0-12-339251-0, MR 1475927

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.