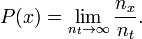Frequentist probability

John Venn

Frequentist probability or frequentism is the standard interpretation of probability; it defines an event's probability as the limit of its relative frequency in a large number of trials.

The development of the frequentist account was motivated by the problems and paradoxes of the previously dominant viewpoint, the classical interpretation. In the classical interpretation, probability was defined in terms of the principle of indifference, based on the natural symmetry of a problem, so, e.g. the probabilities of dice games arise from the natural symmetric 6-sidedness of the cube. This classical interpretation stumbled at any statistical problem that has no natural symmetry for reasoning.

The shift from the classical view to the frequentist view represents a paradigm shift in the progression of statistical thought.^{[citation needed]} This school is often associated with the names of Jerzy Neyman and Egon Pearson who described the logic of statistical hypothesis testing.^{[citation needed]} Other influential figures of the frequentist school include John Venn, R.A. Fisher, and Richard von Mises.^[1]

Definition

In the frequentist interpretation, probabilities are discussed only when dealing with well-defined random experiments.^{[citation needed]} The set of all possible outcomes of a random experiment is called the sample space of the experiment. An event is defined as a particular subset of the sample space to be considered. For any given event, only one of two possibilities may hold: it occurs or it does not. The relative frequency of occurrence of an event, observed in a number of repetitions of the experiment, is a measure of the probability of that event. This is the core conception of probability in the frequentist interpretation.

Thus, if is the total number of trials and 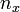is the number of trials where the event occurred, the probability 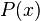of the event occurring will be approximated by the relative frequency as follows:

Clearly, as the number of trials is increased, one might expect the relative frequency to become a better approximation of a "true frequency".

A controversial^{[citation needed]} claim of the frequentist approach is that in the "long run," as the number of trials approaches infinity, the relative frequency will converge exactly to the true probability:^[2]

Such a limit is possible only in theory (e.g. counting the relative fraction of even numbers less than n_t: one may easily compute the limit .) This conflicts with the standard claim^{[citation needed]} that the frequency interpretation is somehow more "objective" than other theories of probability.

Scope

The frequentist interpretation is a philosophical approach to the definition and use of probabilities; it is one of several, and, historically, the earliest to challenge the classical interpretation.^{[citation needed]} It does not claim to capture all connotations of the concept 'probable' in colloquial speech of natural languages.

As an interpretation, it is not in conflict with the mathematical axiomatization of probability theory; rather, it provides guidance for how to apply mathematical probability theory to real-world situations. It offers distinct guidance in the construction and design of practical experiments, especially when contrasted with the Bayesian interpretation. As to whether this guidance is useful, or is apt to mis-interpretation, has been a source of controversy. Particularly when the frequency interpretation of probability is mistakenly assumed to be the only possible basis for frequentist inference. So, for example, a list of mis-interpretations of the meaning of p-values accompanies the article on p-values; controversies are detailed in the article on statistical hypothesis testing. The Jeffreys–Lindley paradox shows how different interpretations, applied to the same data set, can lead to different conclusions about the 'statistical significance' of a result.^{[citation needed]}

As William Feller noted:^[3]

There is no place in our system for speculations concerning the probability that the sun will rise tomorrow. Before speaking of it we should have to agree on an (idealized) model which would presumably run along the lines "out of infinitely many worlds one is selected at random..." Little imagination is required to construct such a model, but it appears both uninteresting and meaningless.

History

The frequentist view was arguably^{[citation needed]} foreshadowed by Aristotle, in Rhetoric,^[4] when he wrote:

the probable is that which for the most part happens^[5]

It was given explicit statement by Robert Leslie Ellis in "On the Foundations of the Theory of Probabilities"^[6] read on 14 February 1842,^[4] (and much later again in "Remarks on the Fundamental Principles of the Theory of Probabilities"^[7]). Antoine Augustin Cournot presented the same conception in 1843, in Exposition de la théorie des chances et des probabilités.^[8]

Perhaps the first elaborate and systematic exposition^{[citation needed]} was by John Venn,^[9] in The Logic of Chance: An Essay on the Foundations and Province of the Theory of Probability (published editions in 1866, 1876, 1888).

Etymology

According to the Oxford English Dictionary, the term 'frequentist' was first used by M. G. Kendall in 1949, to contrast with Bayesians, whom he called "non-frequentists".^[10][11] He observed

3....we may broadly distinguish two main attitudes. One takes probability as 'a degree of rational belief', or some similar idea...the second defines probability in terms of frequencies of occurrence of events, or by relative proportions in 'populations' or 'collectives'; (p. 101)

...

12. It might be thought that the differences between the frequentists and the non-frequentists (if I may call them such) are largely due to the differences of the domains which they purport to cover. (p. 104)

...

I assert that this is not so ... The essential distinction between the frequentists and the non-frequentists is, I think, that the former, in an effort to avoid anything savouring of matters of opinion, seek to define probability in terms of the objective properties of a population, real or hypothetical, whereas the latter do not. [emphasis in original]

Alternative views

The frequentist interpretation does resolve difficulties with the classical interpretation, such as any problem where the natural symmetry of outcomes is not known. It does not address other issues, such as the dutch book. Propensity probability is an alternative physicalist approach.^{[citation needed]}

Notes

References

• P W Bridgman, The Logic of Modern Physics, 1927
• Alonzo Church, The Concept of a Random Sequence, 1940
• Harald Cramér, Mathematical Methods of Statistics, 1946
• William Feller, An introduction to Probability Theory and its Applications, 1957
• P Martin-Löf, On the Concept of a Random Sequence, 1966
• Richard von Mises, Probability, Statistics, and Truth, 1939 (German original 1928)
• Jerzy Neyman, First Course in Probability and Statistics, 1950
• Hans Reichenbach, The Theory of Probability, 1949 (German original 1935)
• Bertrand Russell, Human Knowledge, 1948
• Friedman, C. (1999). "The Frequency Interpretation in Probability". Advances in Applied Mathematics 23 (3): 234–174. doi:10.1006/aama.1999.0653.  PS