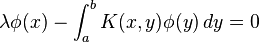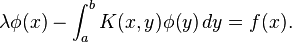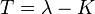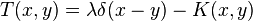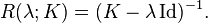Fredholm alternative
In mathematics, the Fredholm alternative, named after Ivar Fredholm, is one of Fredholm's theorems and is a result in Fredholm theory. It may be expressed in several ways, as a theorem of linear algebra, a theorem of integral equations, or as a theorem on Fredholm operators. Part of the result states that a non-zero complex number in the spectrum of a compact operator is an eigenvalue.
Linear algebra
If V is an n-dimensional vector space and  is a linear transformation, then exactly one of the following holds:
is a linear transformation, then exactly one of the following holds:
- For each vector v in V there is a vector u in V so that
 . In other words: T is surjective (and so also bijective, since V is finite-dimensional).
. In other words: T is surjective (and so also bijective, since V is finite-dimensional).  .
.
A more elementary formulation, in terms of matrices, is as follows. Given an m×n matrix A and a m×1 column vector b, exactly one of the following must hold:
- Either: A x = b has a solution x
- Or: AT y = 0 has a solution y with yTb ≠ 0.
In other words, A x = b has a solution  if and only if for any y s.t. AT y = 0, yTb = 0
if and only if for any y s.t. AT y = 0, yTb = 0 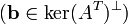 .
.
Integral equations
Let  be an integral kernel, and consider the homogeneous equation, the Fredholm integral equation,
be an integral kernel, and consider the homogeneous equation, the Fredholm integral equation,
and the inhomogeneous equation
The Fredholm alternative states that, for any non-zero fixed complex number 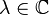 , either the first equation has a non-trivial solution, or the second equation has a solution for all
, either the first equation has a non-trivial solution, or the second equation has a solution for all  .
.
A sufficient condition for this theorem to hold is for  to be square integrable on the rectangle
to be square integrable on the rectangle ![[a,b]\times [a,b]](/2014-wikipedia_en_all_02_2014/I/media/3/6/6/a/366a95831770edb4c381dab2095cf2ff.png) (where a and/or b may be minus or plus infinity).
(where a and/or b may be minus or plus infinity).
Functional analysis
Results on the Fredholm operator generalize these results to vector spaces of infinite dimensions, Banach spaces.
Correspondence
Loosely speaking, the correspondence between the linear algebra version, and the integral equation version, is as follows: Let
or, in index notation,
with  the Dirac delta function. Here, T can be seen to be an linear operator acting on a Banach space V of functions
the Dirac delta function. Here, T can be seen to be an linear operator acting on a Banach space V of functions 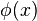 , so that
, so that
is given by
with  given by
given by
In this language, the integral equation alternatives are seen to correspond to the linear algebra alternatives.
Alternative
In more precise terms, the Fredholm alternative only applies when K is a compact operator. From Fredholm theory, smooth integral kernels are compact operators. The Fredholm alternative may be restated in the following form: a nonzero 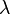 is either an eigenvalue of K, or it lies in the domain of the resolvent
is either an eigenvalue of K, or it lies in the domain of the resolvent
See also
References
- E.I. Fredholm, "Sur une classe d'equations fonctionnelles", Acta Math., 27 (1903) pp. 365–390.
- A. G. Ramm, "A Simple Proof of the Fredholm Alternative and a Characterization of the Fredholm Operators", American Mathematical Monthly, 108 (2001) p. 855.
- Khvedelidze, B.V. (2001), "Fredholm theorems for integral equations", in Hazewinkel, Michiel, Encyclopedia of Mathematics, Springer, ISBN 978-1-55608-010-4
- Weisstein, Eric W., "Fredholm Alternative", MathWorld.