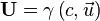Four-velocity
In physics, in particular in special relativity and general relativity, a four-velocity is a four-vector (vector in four-dimensional spacetime) that replaces velocity (a three-dimensional vector).
Events are described in time and space, together forming four-dimensional spacetime. The history of an object traces a curve in spacetime, called its world line, which may be parametrized by the proper time of the object. The four-velocity is the rate of change of four-position with respect to the proper time along the curve. The velocity, in contrast, is the rate of change of the position in (three-dimensional) space of the object, as seen by an inertial observer, with respect to the observer's time.
A four-velocity is thus the normalized future-directed timelike tangent vector to a world line, and is a contravariant vector. Though it is a vector, addition of two four-velocities does not yield a four-velocity: the space of four-velocities is not itself a vector space.
The magnitude of an object's four-velocity is always equal to c, the speed of light. For an object at rest (with respect to the coordinate system) its four-velocity points in the direction of the time coordinate.
Velocity
The path of an object in three-dimensional space (in an inertial frame) may be expressed in terms of three coordinate functions 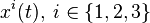 of time
of time 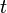 :
:
where the  denote the three spatial coordinates of the object at time t.
denote the three spatial coordinates of the object at time t.
The components of the velocity  (tangent to the curve) at any point on the world line are
(tangent to the curve) at any point on the world line are
Theory of relativity
In Einstein's theory of relativity, the path of an object moving relative to a particular frame of reference is defined by four coordinate functions  (where
(where 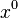 denotes the time coordinate multiplied by c), each function depending on one parameter
denotes the time coordinate multiplied by c), each function depending on one parameter  , called its proper time.
, called its proper time.
Time dilation
From time dilation, we know that
where  is the Lorentz factor, which is defined as:
is the Lorentz factor, which is defined as:
and u is the Euclidean norm of the velocity vector  :
:
 .
.
Definition of the four-velocity
The four-velocity is the tangent four-vector of a world line.
The four-velocity at any point of world line  is defined as:
is defined as:
where  is the four-position and
is the four-position and  is the proper time.
is the proper time.
The four-velocity defined here using the proper time of an object does not exist for world lines for objects such as photons travelling at the speed of light; nor is it defined for tachyonic world lines, where the tangent vector is spacelike.
Components of the four-velocity
The relationship between the time t and the coordinate time  is given by
is given by
Taking the derivative with respect to the proper time 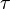 , we find the
, we find the  velocity component for μ = 0:
velocity component for μ = 0:
Using the chain rule, for 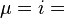 1, 2, 3, we have
1, 2, 3, we have
where we have used the relationship
Thus, we find for the four-velocity 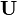 :
:
In terms of the yardsticks (and synchronized clocks) associated
with a particular slice of flat spacetime, the three spacelike
components of four-velocity define a traveling object's
proper velocity  i.e.
the rate at which distance is covered in the reference map frame
per unit proper time elapsed on clocks traveling with the object.
i.e.
the rate at which distance is covered in the reference map frame
per unit proper time elapsed on clocks traveling with the object.
See also
- four-vector, four-acceleration, four-momentum, four-force.
- Special Relativity, Calculus, Derivative.
- Algebra of physical space
- Congruence (general relativity)
References
- Einstein, Albert; translated by Robert W. Lawson (1920). Relativity: The Special and General Theory. New York: Original: Henry Holt, 1920; Reprinted: Prometheus Books, 1995.
- Rindler, Wolfgang (1991). Introduction to Special Relativity (2nd). Oxford: Oxford University Press. ISBN 0-19-853952-5.