Fσ set

From Wikipedia, the free encyclopedia

In mathematics, an F_σ set (said F-sigma set) is a countable union of closed sets. The notation originated in France with F for fermé (French: closed) and σ for somme (French: sum, union).

In metrizable spaces, every open set is an F_σ set. The complement of an F_σ set is a G_δ set. In a metrizable space, any closed set is a G_δ set.

The union of countably many F_σ sets is an F_σ set, and the intersection of finitely many F_σ sets is an F_σ set. F_σ is the same as 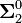${\mathbf {\Sigma }}_{2}^{0}$ in the Borel hierarchy.

Examples

Each closed set is an F_σ set.

The set ${\mathbb {Q}}$ of rationals is an F_σ set. The set ${\mathbb {R}}\setminus {\mathbb {Q}}$ of irrationals is not a F_σ set.

In a Tychonoff space, each countable set is an F_σ set, because a point 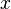${x}$ is closed.

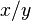For example, the set $A$ of all points $(x,y)$ in the Cartesian plane such that $x/y$ is rational is an F_σ set because it can be expressed as the union of all the lines passing through the origin with rational slope:

$A=\bigcup _{{r\in {\mathbb {Q}}}}\{(ry,y)\mid y\in {\mathbb {R}}\},$

where ${\mathbb {Q}}$ , is the set of rational numbers, which is a countable set.

Fσ set

Examples

See also