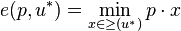Expenditure function

From Wikipedia, the free encyclopedia

In microeconomics, the expenditure function describes the minimum amount of money an individual needs to achieve some level of utility, given a utility function and prices.

Formally, if there is a utility function u that describes preferences over L commodities, the expenditure function

e(p,u^{*}):{\textbf R}_{+}^{L}\times {\textbf R}\rightarrow {\textbf R}

says what amount of money is needed to achieve a utility u^{*} if prices are set by p. This function is defined by

e(p,u^{*})=\min _{{x\in \geq (u^{*})}}p\cdot x

where

\geq (u^{*})=\{x\in {\textbf R}_{+}^{L}:u(x)\geq u^{*}\}

is the set of all bundles that give utility at least as good as u^{*}.

See also

References

  • Andreu Mas-Colell, Michael D. Whinston and Jerry R. Green Microeconomic Theory, 2007, ISBN 0-19-510268-1, pp. 59-60, The Expenditure Function
This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.