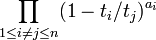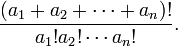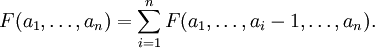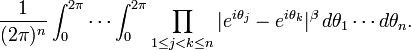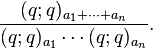Dyson conjecture
In mathematics, the Dyson conjecture (Freeman Dyson 1962) is a conjecture about the constant term of certain Laurent polynomials, proved by Wilson and Gunson. Andrews generalized it to the q-Dyson conjecture, proved by Zeilberger and Bressoud and sometimes called the Zeilberger–Bressoud theorem. Macdonald generalized it further to more general root systems with the Macdonald constant term conjecture, proved by Cherednik.
Dyson conjecture
The Dyson conjecture states that the Laurent polynomial
has constant term
The conjecture was first proved independently by Wilson (1962) and Gunson (1962). Good (1970) later found a short proof, by observing that the Laurent polynomials, and therefore their constant terms, satisfy the recursion relations
The case n = 3 of Dyson's conjecture follows from the Dixon identity.
Sills & Zeilberger (2006) and (Sills 2006) used a computer to find expressions for non-constant coefficients of Dyson's Laurent polynomial.
Dyson integral
When all the values ai are equal to β/2, the constant term in Dyson's conjecture is the value of Dyson's integral
Dyson's integral is a special case of Selberg's integral after a change of variable and has value
which gives another proof of Dyson's conjecture in this special case.
q-Dyson conjecture
Andrews (1975) found a q-analog of Dyson's conjecture, stating that the constant term of
is
Here (a;q)n is the q-Pochhammer symbol. This conjecture reduces to Dyson's conjecture for q=1, and was proved by Zeilberger & Bressoud (1985).
Macdonald conjectures
Macdonald (1982) extended the conjecture to arbitrary finite or affine root systems, with Dyson's original conjecture corresponding to the case of the An−1 root system and Andrews's conjecture corresponding to the affine An−1 root system. Macdonald reformulated these conjectures as conjectures about the norms of Macdonald polynomials. Macdonald's conjectures were proved by (Cherednik 1995) using doubly affine Hecke algebras.
Macdonald's form of Dyson's conjecture for root systems of type BC is closely related to Selberg's integral.
References
- Andrews, George E. (1975), "Problems and prospects for basic hypergeometric functions", Theory and application of special functions (Proc. Advanced Sem., Math. Res. Center, Univ. Wisconsin, Madison, Wis., 1975), Boston, MA: Academic Press, pp. 191–224, MR 0399528
- Cherednik, I. (1995), "Double Affine Hecke Algebras and Macdonald's Conjectures", The Annals of Mathematics 141 (1): 191–216, doi:10.2307/2118632, JSTOR 2118632
- Dyson, Freeman J. (1962), "Statistical theory of the energy levels of complex systems. I", Journal of Mathematical Physics 3: 140–156, doi:10.1063/1.1703773, ISSN 0022-2488, MR 0143556
- Good, I. J. (1970), "Short proof of a conjecture by Dyson", Journal of Mathematical Physics 11 (6): 1884, doi:10.1063/1.1665339, ISSN 0022-2488, MR 0258644
- Gunson, J. (1962), "Proof of a conjecture by Dyson in the statistical theory of energy levels", Journal of Mathematical Physics 3 (4): 752–753, doi:10.1063/1.1724277, ISSN 0022-2488, MR 0148401
- Macdonald, I. G. (1982), "Some conjectures for root systems", SIAM Journal on Mathematical Analysis 13 (6): 988–1007, doi:10.1137/0513070, ISSN 0036-1410, MR 674768
- Sills, Andrew V. (2006), "Disturbing the Dyson conjecture, in a generally GOOD way", Journal of Combinatorial Theory. Series A 113 (7): 1368–1380, doi:10.1016/j.jcta.2005.12.005, ISSN 1096-0899, MR 2259066
- Sills, Andrew V.; Zeilberger, Doron (2006), "Disturbing the Dyson conjecture (in a GOOD way)", Experimental Mathematics 15 (2): 187–191, doi:10.1080/10586458.2006.10128959, ISSN 1058-6458, MR 2253005
- Wilson, Kenneth G. (1962), "Proof of a conjecture by Dyson", Journal of Mathematical Physics 3 (5): 1040–1043, doi:10.1063/1.1724291, ISSN 0022-2488, MR 0144627
- Zeilberger, Doron; Bressoud, David M. (1985), "A proof of Andrews' q-Dyson conjecture", Discrete Mathematics 54 (2): 201–224, doi:10.1016/0012-365X(85)90081-0, ISSN 0012-365X, MR 791661