Double-sideband suppressed-carrier transmission

From Wikipedia, the free encyclopedia

Double-sideband suppressed-carrier transmission (DSB-SC) is transmission in which frequencies produced by amplitude modulation (AM) are symmetrically spaced above and below the carrier frequency and the carrier level is reduced to the lowest practical level, ideally being completely suppressed.

In the DSB-SC modulation, unlike in AM, the wave carrier is not transmitted; thus, much of the power is distributed between the sidebands, which implies an increase of the cover in DSB-SC, compared to AM, for the same power used.

DSB-SC transmission is a special case of double-sideband reduced carrier transmission. It is used for radio data systems.

Spectrum

DSB-SC is basically an amplitude modulation wave without the carrier, therefore reducing power waste, giving it a 50% efficiency. This is an increase compared to normal AM transmission (DSB), which has a maximum efficiency of 33.333%, since 2/3 of the Power is in the Carrier which carries NO intelligence, and each Sideband carries the SAME Information. Single_Side_Band (SSB) Suppressed_Carrier is 100% efficient.

Spectrum plot of an DSB-SC signal:

Generation

DSB-SC is generated by a mixer. This consists of a message signal combined with the frequency carrier.

$\underbrace {V_{m}\cos \left(\omega _{m}t\right)}_{{{\mbox{Audio}}}}\times \underbrace {V_{c}\cos \left(\omega _{c}t\right)}_{{{\mbox{Carrier}}}}={\frac {V_{m}V_{c}}{2}}\left[\underbrace {\cos \left(\left(\omega _{c}+\omega _{m}\right)t\right)}_{{{\mbox{USB}}}}+\underbrace {\cos \left(\left(\omega _{c}-\omega _{m}\right)t\right)}_{{{\mbox{LSB}}}}\right]$

Demodulation

For demodulation, the audio frequency and the carrier frequency must be exact. Otherwise, distortion will occur. DSB-SC can be demodulated if modulation index is less than unity.

How it works

This is best shown graphically. Below is a message signal that one may wish to modulate onto a carrier, consisting of a couple of sinusoidal components.

The equation for this message signal is $s(t)={\frac {1}{2}}\cos \left(2\pi 800t\right)-{\frac {1}{2}}\cos \left(2\pi 1200t\right)$ .

The carrier, in this case, is a plain 5 kHz (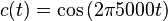 $c(t)=\cos \left(2\pi 5000t\right)$ ) sinusoid—pictured below.

The modulation is performed by multiplication in the time domain, which yields a 5 kHz carrier signal, whose amplitude varies in the same manner as the message signal.

$x(t)=\underbrace {\cos \left(2\pi 5000t\right)}_{{\mbox{Carrier}}}\times \underbrace {\left[{\frac {1}{2}}\cos \left(2\pi 800t\right)-{\frac {1}{2}}\cos \left(2\pi 1200t\right)\right]}_{{\mbox{Message Signal}}}$

The name "suppressed carrier" comes about because the carrier signal component is suppressed—it does not appear in the output signal. This is apparent when the spectrum of the output signal is viewed:

References

This article incorporates public domain material from the General Services Administration document "Federal Standard 1037C" (in support of MIL-STD-188).

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.