Discounted utility
Discounted utility is an economics term in which economists, accountants, underwriters, and other financial analysts include the future discounted value of a good in its present value. This can be thought of as the idea of impatience, or the valuing of future enjoyment less than present enjoyment.
Such value calculations take the form:
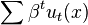
where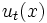 
is the value of some choice 
at time 
. 
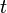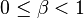, so that 
can be interpreted as a 'discounting factor'. In financial analysis, may represent lost opportunities, such as the risk-free rate of return on a government, municipal, or corporate bond.
In economics, however, where 
represents so-called utility, the interpretation of is less straightforward. Sometimes it is explained as the degree of a person's patience. Given the interpretation of economic agents as rational, this exempts time-valuations from rationality judgments, so that someone who spends and borrows voraciously is just as rational as someone who spends and saves moderately, or as someone who hoards his wealth and never spends it.
Some formulations treat not as a constant, but as a function 
that itself varies over time, for example in models which use the concept of hyperbolic discounting. This view is consistent with empirical observations that humans display inconsistent time preferences. For example, experiments by Tversky and Kahneman showed that the same people who would choose 1 candy bar now over 2 candy bars tomorrow, would choose 2 candy bars 101 days from now over 1 candy bar 100 days from now. (This is inconsistent because if the same question were posed 100 days from now, the person would ostensibly again choose 1 candy bar immediately instead of 2 candy bars the next day.)
Despite arguments about how should be interpreted, the basic idea is that all other things equal, the agent prefers to have something now as opposed to later (hence 
).