Decagonal prism
From Wikipedia, the free encyclopedia
| Uniform Decagonal prism | |
|---|---|
 | |
| Type | Prismatic uniform polyhedron |
| Elements | F = 12, E = 30, V = 20 (χ = 2) |
| Faces by sides | 10{4}+2{10} |
| Schläfli symbol | t{2,10} or {10}x{} |
| Wythoff symbol | 2 10 | 2 2 2 5 | |
| Coxeter diagrams | |
| Symmetry | D10h, [10,2], (*10.2.2), order 40 |
| Rotation group | D10, [10,2]+, (10.2.2), order 20 |
| References | U76(h) |
| Dual | Decagonal dipyramid |
| Properties | convex, zonohedron |
 Vertex figure 4.4.10 | |
In geometry, the decagonal prism is the eighth in the infinite set of prisms, formed by ten square side faces and two regular decagon caps. With twelve faces, it is one of many nonregular dodecahedra. The decagonal prism has 12 faces, 30 edges, and 20 vertices. If faces are all regular, it is a semiregular or prismatic uniform polyhedron.
Uses
The decagonal prism exists as cells in two four-dimensional uniform polychora:
| Runcitruncated 120-cell |
Omnitruncated 120-cell |
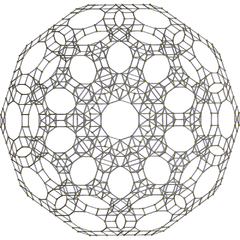 |
 |
Related polyhedra
| Symmetry | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
|---|---|---|---|---|---|---|---|---|---|---|
| [2n,2] [n,2] [2n,2+] [2n+,2] |
||||||||||
| Image | ||||||||||
| As spherical polyhedra | ||||||||||
| Image | ||||||||||
External links
This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.