Coxeter element

From Wikipedia, the free encyclopedia

Not to be confused with Longest element of a Coxeter group.

In mathematics, the Coxeter number h is the order of a Coxeter element of an irreducible Coxeter group, hence also of a root system or its Weyl group. It is named after H.S.M. Coxeter.^[1]

Definitions

Warning: this article assumes a finite Coxeter group. For infinite Coxeter groups, there are multiple conjugacy classes of Coxeter elements, and they have infinite order.

There are many different ways to define the Coxeter number h of an irreducible root system.

A Coxeter element is a product of all simple reflections. The product depends on the order in which they are taken, but different orderings produce conjugate elements, which have the same order.

The Coxeter number is the number of roots divided by the rank.
The Coxeter number is the order of a Coxeter element; note that conjugate elements have the same order.
If the highest root is ∑m_iα_i for simple roots α_i, then the Coxeter number is 1 + ∑m_i
The dimension of the corresponding Lie algebra is n(h + 1), where n is the rank and h is the Coxeter number.
The Coxeter number is the highest degree of a fundamental invariant of the Weyl group acting on polynomials.
The Coxeter number is given by the following table:

Coxeter group		Coxeter number h	Dual Coxeter number	Degrees of fundamental invariants
A_n	...	n + 1	n + 1	2, 3, 4, ..., n + 1
B_n	...	2n	2n − 1	2, 4, 6, ..., 2n
C_n	...	2n	n + 1	2, 4, 6, ..., 2n
D_n	...	2n − 2	2n − 2	n; 2, 4, 6, ..., 2n − 2
E₆		12	12	2, 5, 6, 8, 9, 12
E₇		18	18	2, 6, 8, 10, 12, 14, 18
E₈		30	30	2, 8, 12, 14, 18, 20, 24, 30
F₄		12	9	2, 6, 8, 12
G₂ = I₂(6)		6	4	2, 6
H₃		10		2, 6, 10
H₄		30		2, 12, 20, 30
I₂(p)		p		2, p

The invariants of the Coxeter group acting on polynomials form a polynomial algebra whose generators are the fundamental invariants; their degrees are given in the table above. Notice that if m is a degree of a fundamental invariant then so is h + 2 − m.

The eigenvalues of a Coxeter element are the numbers e^{2πi(m − 1)/h} as m runs through the degrees of the fundamental invariants. Since this starts with m = 2, these include the primitive hth root of unity, ζ_h = e^2πi/h, which is important in the Coxeter plane, below.

Coxeter elements

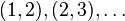Coxeter elements of $A_{{n-1}}\cong S_{n}$ , considered as the symmetric group on n elements, are n-cycles: for simple reflections the adjacent transpositions $(1,2),(2,3),\dots$ , a Coxeter element is the n-cycle $(1,2,3,\dots ,n)$ .^[2]

The dihedral group Dih_m is generated by two reflections that form an angle of $2\pi /2m$ , and thus their product is a rotation by $2\pi /m$ .

Coxeter plane

Projection of E₈ root system onto Coxeter plane, showing 30-fold symmetry.

For a given Coxeter element w, there is a unique plane P on which w acts by rotation by 2π/h. This is called the Coxeter plane and is the plane on which P has eigenvalues e^2πi/h and e^−2πi/h = e^{2πi(h−1)/h}.^[3] This plane was first systematically studied in (Coxeter 1948),^[4] and subsequently used in (Steinberg 1959) to provide uniform proofs about properties of Coxeter elements.^[4]

The Coxeter plane is often used to draw diagrams of higher-dimensional polytopes and root systems – the vertices and edges of the polytope, or roots (and some edges connecting these) are orthogonally projected onto the Coxeter plane, yielding a Petrie polygon with h-fold rotational symmetry.^[5] For root systems, no root maps to zero, corresponding to the Coxeter element not fixing any root or rather axis (not having eigenvalue 1 or −1), so the projections of orbits under w form h-fold circular arrangements^[5] and there is an empty center, as in the E₈ diagram at above right. For polytopes, a vertex may map to zero, as depicted below. Projections onto the Coxeter plane are depicted below for the Platonic solids.

Petrie polygons of the Platonic solids, showing 4-fold, 6-fold, and 10-fold symmetry, corresponding to the Coxeter lengths of A₃, BC₃, and H₃.

Notes

↑ Coxeter, Harold Scott Macdonald; Chandler Davis, Erlich W. Ellers (2006), The Coxeter Legacy: Reflections and Projections, AMS Bookstore, p. 112, ISBN 978-0-8218-3722-1 Cite uses deprecated parameters (help)
↑ (Humphreys 1992, p. 75)
↑ (Humphreys 1992, Section 3.17, "Action on a Plane", pp. 76–78)
↑ 4.0 4.1 (Reading 2010, p. 2)
↑ 5.0 5.1 (Stembridge 2007)

References

Coxeter, H. S. M. (1948), Regular Polytopes, Methuen and Co.
Steinberg, R. (June 1959), "Finite Reflection Groups", Transactions of the American Mathematical Society 91 (3): 493–504, doi:10.1090/S0002-9947-1959-0106428-2, ISSN 0002-9947, JSTOR 1993261
Hiller, Howard Geometry of Coxeter groups. Research Notes in Mathematics, 54. Pitman (Advanced Publishing Program), Boston, Mass.-London, 1982. iv+213 pp. ISBN 0-273-08517-4
Humphreys, James E. (1992), Reflection Groups and Coxeter Groups, Cambridge University Press, pp. 74–76 (Section 3.16, Coxeter Elements), ISBN 978-0-521-43613-7
Stembridge, John (April 9, 2007), Coxeter Planes
Stekolshchik, R. (2008), Notes on Coxeter Transformations and the McKay Correspondence, Springer Monographs in Mathematics, doi:10.1007/978-3-540-77398-3, ISBN 978-3-540-77398-6
Reading, Nathan (2010), "Noncrossing Partitions, Clusters and the Coxeter Plane", Séminaire Lotharingien de Combinatoire B63b: 32

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.