Combination tone

From Wikipedia, the free encyclopedia
Sum and difference tones of A220 and unison, just perfect fifth, and octave. Hz marked.  Play (help·info)

A combination tone, also called a sum tone or a difference tone (also occasionally resultant tone), can be any of at least three similar psychoacoustic phenomena. When two tones are played simultaneously, a listener can sometimes perceive an additional tone whose frequency is a sum or difference of the two frequencies. The discovery of some of these phenomena is credited to the violinist Giuseppe Tartini, and so the tones are also called Tartini tones.

Explanation

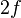One way a difference tone can be heard is when two tones with fairly complete sets of harmonics make a just fifth. This can be explained as an example of the missing fundamental phenomenon.[1] If f is the missing fundamental frequency, then 2f would be the frequency of the lower tone, and its harmonics would be 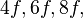4f,6f,8f, etc. Since a fifth corresponds to a frequency ratio of 2:3, the higher tone and its harmonics would then be 3f,6f,9f, etc. When both tones are sounded, there are components with frequencies of 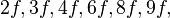2f,3f,4f,6f,8f,9f, etc. The missing fundamental is heard because so many of these components refer to it.

The specific phenomenon that Tartini discovered was physical. Sum and difference tones are thought to be caused sometimes by the non-linearity of the inner ear. This causes intermodulation distortion of the various frequencies which enter the ear. They are combined linearly, generating relatively faint components with frequencies equal to the sums and differences of whole multiples of the original frequencies. Any components which are heard are usually lower, with the most commonly heard frequency being just the difference tone, 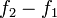f_{2}-f_{1}, though this may be a consequence of the other phenomena. Although much less common, the following frequencies may also be heard:

2f_{1}-f_{2},3f_{1}-2f_{2},\ldots ,f_{1}-k(f_{2}-f_{1})

For a time it was thought that the inner ear was solely responsible whenever a sum or difference tone was heard. However, experiments show evidence that even when using headphones providing a single pure tone to each ear separately, listeners may still hear a difference tone[citation needed]. Since the peculiar, non-linear physics of the ear doesn't come into play in this case, it is thought that this must be a separate, neural phenomenon. Compare binaural beats.

Heinz Bohlen proposed what is now known as the Bohlen–Pierce scale on the basis of combination tones,[2] as well as the 833 cents scale.

alt text
 Play (help·info)

Resultant tone

In pipe organs, a resultant tone is the sound of a combination of organ pipes that allows the listener to perceive a lower pitch.[3]

This is done by having two pipes, one pipe of the note being played, and another harmonically related, typically at its fifth, being sounded at the same time. The result is a pitch at a common subharmonic of the pitches played (one octave below the first pitch when the second is the fifth, 3:2, two octaves below when the second is the major third, 5:4). This effect is useful especially in the lowest ranks of the pipe organ where cost or space could prohibit having a rank of such low pitch. For example, a 64' pipe would be costly and take up at least 32' of space (if capped) for each pipe. Using a resultant tone for such low pitches would eliminate the cost and space factor, but would not sound as full as a true 64' pipe.

This effect is most often used in the lowest octave of the organ only.

See also

References

  1. ↑ Beament, James (2001). How We Hear Music, . The Boydell Press. ISBN 0-85115-813-7.
  2. ↑ Max V. Mathews and John R. Pierce (1989). "The Bohlen–Pierce Scale", p.167. Current Directions in Computer Music Research, Max V. Mathews and John R. Pierce, eds. MIT Press.
  3. ↑ James Ingall Wedgwood (1907). A Comprehensive Dictionary of Organ Stops: English and foreign, ancient and modern: practical, theoretical, historical, aesthetic, etymological, phonetic (2nd ed.). G. Schirmer. p. 1. 

Further reading

External links

Engineering
Psychoacoustics
Acousticians
Related topics
This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.