Cohen ring

From Wikipedia, the free encyclopedia

Not to be confused with Cohen–Macaulay ring.

In algebra, a Cohen ring is a field or a complete discrete valuation ring of mixed characteristic 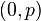$(0,p)$ whose maximal ideal is generated by p. Cohen rings are used in the Cohen structure theorem for complete Noetherian local rings.

See also

Norm field

References

Cohen, I. S. (1946), "On the structure and ideal theory of complete local rings", Transactions of the American Mathematical Society 59: 54–106, ISSN 0002-9947, MR 0016094 Cohen's paper was written when "local ring" meant what is now called a "Noetherian local ring".
Grothendieck, Alexandre; Dieudonné, Jean (1964). "Éléments de géométrie algébrique: IV. Étude locale des schémas et des morphismes de schémas, Première partie". Publications Mathématiques de l'IHÉS 20: 5–259. MR 0173675.

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.