Cantic cubic honeycomb

From Wikipedia, the free encyclopedia
Cantic cubic honeycomb
 
TypeUniform honeycomb
Schläfli symbolh2{4,3,4}
Coxeter-Dynkin diagram =
=
Cellst{3,4}
r{4,3}
t{3,3}
Vertex figure
Coxeter groups[4,31,1], {{\tilde {B}}}_{3}
[3[4]], {{\tilde {A}}}_{3}
Symmetry groupFm3m (225)
Dual half oblate octahedrille
Propertiesvertex-transitive

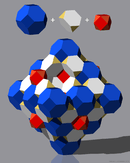
The cantic cubic honeycomb or truncated half cubic honeycomb is a uniform space-filling tessellation (or honeycomb) in Euclidean 3-space. It is composed of truncated octahedra, cuboctahedra and truncated tetrahedra in a ratio of 1:1:2. Its vertex figure is a rectangular pyramid.

John Horton Conway calls this honeycomb a truncated tetraoctahedrille, and its dual half oblate octahedrille.

Symmetry

It has two different uniform constructions. The {{\tilde {A}}}_{3} construction can be seen with alternately colored truncated tetrahedra.

Symmetry [4,31,1], {{\tilde {B}}}_{3}
=<[3[4]]>		 [3[4]], {{\tilde {A}}}_{3}
Space group Fm3m (225) F43m (216)
Coloring
Coxeter = =
Vertex figure

It is related to the cantellated cubic honeycomb. Rhombicuboctahedra are reduced to truncated octahedra, and cubes are reduced to truncated tetrahedra.


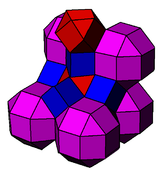cantellated cubic
Cantic cubic

, ,
rr{4,3}, r{4,3}, {4,3}		 , ,
t{3,4}, r{4,3}, t{3,3}

Related honeycombs

Space
group		 Fibrifold Extended
symmetry		 Extended
diagram		 Order Honeycombs
Pm3m
(221)		 4−:2 [4,3,4] ×1 1, 2, 3, 4,
5, 6
Fm3m
(225)		 2−:2 [1+,4,3,4]
= [4,31,1]
= 		Half 7, 11, 12, 13
I43m
(217)		 4o:2 [[(4,3,4,2+)]] Half × 2 (7),
Fd3m
(227)		 2+:2 [[1+,4,3,4,1+]]
= [[3[4]]]
= 		Quarter × 2 10,
Im3m
(229)		 8o:2 [[4,3,4]] ×2

(1), 8, 9

The [4,31,1], , Coxeter group generates 9 permutations of uniform tessellations, 4 with distinct geometry including the alternated cubic honeycomb.

Space
group		 Fibrifold Extended
symmetry		 Extended
diagram		 Order Honeycombs
Fm3m
(225)		 2−:2 [4,31,1]
= [4,3,4,1+]
= 		×1 1, 2, 3, 4
Fm3m
(225)		 2−:2 <[1+,4,31,1]>
= <[3[4]]>
= 		×2 (1), (3)
Pm3m
(221)		 4−:2 <[4,31,1]> ×2

5, 6, 7, (6), 9, 10, 11

This honeycomb is one of five distinct uniform honeycombs[1] constructed by the {{\tilde {A}}}_{3} Coxeter group. The symmetry can be multiplied by the symmetry of rings in the Coxeter–Dynkin diagrams:

Space
group		 Fibrifold Square
symmetry		 Extended
symmetry		 Extended
diagram		 Extended
order		 Honeycomb diagrams
F43m
(216)		 1o:2 a1 [3[4]] ×1 (None)
Fd3m
(227)		 2+:2 p2 [[3[4]]]
= 		×2  3
Fm3m
(225)		 2−:2 d2 <[3[4]]>
= [4,3,31,1]
= 		×2  1, 2
Pm3m
(221)		 4−:2 d4 [2[3[4]]]
= [4,3,4]
= 		×4  4
Im3m
(229)		 8o:2 r8 [4[3[4]]]
= [[4,3,4]]
= 		×8  5,  (*)

See also

References

Wikimedia Commons has media related to Truncated alternated cubic honeycomb.
  1. ↑ , A000029 6-1 cases, skipping one with zero marks
  • John H. Conway, Heidi Burgiel, Chaim Goodman-Strauss, (2008) The Symmetries of Things, ISBN 978-1-56881-220-5 (Chapter 21, Naming the Archimedean and Catalan polyhedra and tilings, Architectonic and Catoptric tessellations, p 292-298, includes all the nonprismatic forms)
  • George Olshevsky, Uniform Panoploid Tetracombs, Manuscript (2006) (Complete list of 11 convex uniform tilings, 28 convex uniform honeycombs, and 143 convex uniform tetracombs)
  • Branko Grünbaum, Uniform tilings of 3-space. Geombinatorics 4(1994), 49 - 56.
  • Norman Johnson Uniform Polytopes, Manuscript (1991)
  • Williams, Robert (1979). The Geometrical Foundation of Natural Structure: A Source Book of Design. Dover Publications, Inc. ISBN 0-486-23729-X. 
  • Critchlow, Keith (1970). Order in Space: A design source book. Viking Press. ISBN 0-500-34033-1. 
  • Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6
    • (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I, [Math. Zeit. 46 (1940) 380-407, MR 2,10] (1.9 Uniform space-fillings)
  • A. Andreini, Sulle reti di poliedri regolari e semiregolari e sulle corrispondenti reti correlative (On the regular and semiregular nets of polyhedra and on the corresponding correlative nets), Mem. Società Italiana della Scienze, Ser.3, 14 (1905) 75–129.
  • D. M. Y. Sommerville, An Introduction to the Geometry of n Dimensions. New York, E. P. Dutton, 1930. 196 pp. (Dover Publications edition, 1958) Chapter X: The Regular Polytopes
  • Richard Klitzing, 3D Euclidean Honeycombs, x3x3o *b4o - tatoh - O25
  • Uniform Honeycombs in 3-Space: 13-Tatoh
This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.